I don't understand the embolded phrase below. I'm unschooled at physics and photography.
Starburst and Sunstar Effects
Starbursts, also called sunstars, are beautiful elements that you’ll find in certain photographs. Despite the odd names – one, a type of candy; the other, a type of starfish – I always try to capture them in my landscape photos. Here’s an example:
The sunbeams in this photo are purely a result of my aperture (in this case, f/16).
How does this work? Essentially, for every aperture blade in your lens, you’ll end up with a sunbeam. This only happens if you photograph a small, bright point of light, such as the sun when it is partly blocked. This is fairly common in landscape photography. If you want the strongest possible starburst, use a small aperture. When the sun is in my photo, I typically set f/16 purely to capture this effect.
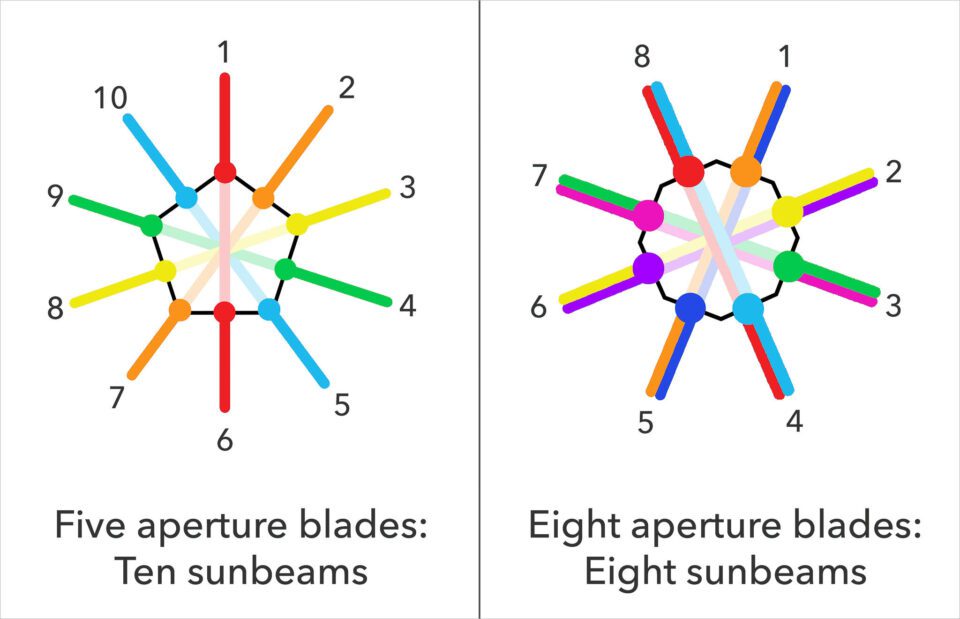
Also, the starburst effect looks different from lens to lens. It all depends upon your aperture blades. If your lens has six aperture blades, you’ll get six sunbeams. If your lens has eight aperture blades, you’ll get eight sunbeams. And, if your lens has nine aperture blades, you’ll get eighteen sunbeams.
Wait, what?
That’s no typo. For lenses with an odd number of aperture blades, you’ll get twice as many sunbeams. Why is that?
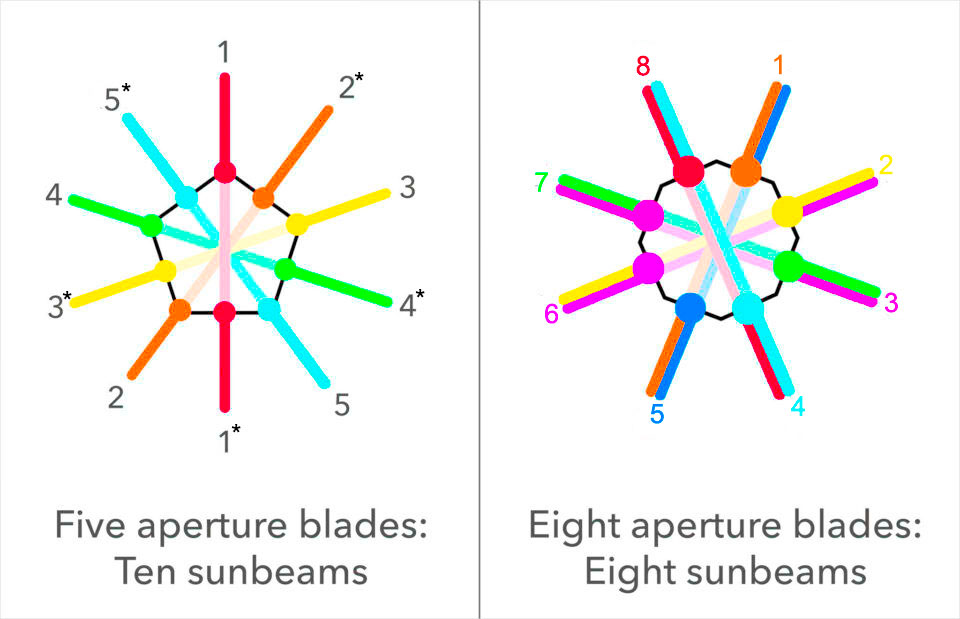
It sounds strange, but the reason is actually quite simple. In lenses with an even number of aperture blades (and a fully symmetrical design), half of the sunbeams will overlap the other half. So, you don’t see all of them in your final photo.
Here’s a diagram to show what I mean:
When you have an even number of aperture blades, the sunbeams will overlap.
Most Nikon lenses have seven or nine aperture blades, resulting in 14 and 18 sunbeams respectively. Most Canon lenses have eight aperture blades, resulting in eight sunbeams. I took the photo above using the Nikon 20mm f/1.8G lens, which has 7 aperture blades. That’s why the image has 14 sunbeams.