I'm wondering how sensor sizes are calculated. The Sony RX100 reportedly has a sensor size of 1" which is 13.2 x 8.8 mm according to the press release and various sites.
How does that work? That's a pretty small inch.
The Wikipedia page on sensor sizes has this chart: 
which doesn't really help. (The 1" format is labelled Nikon CX. Chart by Moxfyre under the CC-BY-SA license.)
If full frame/35 mm refers to the horizontal size, what does 1" refer to?
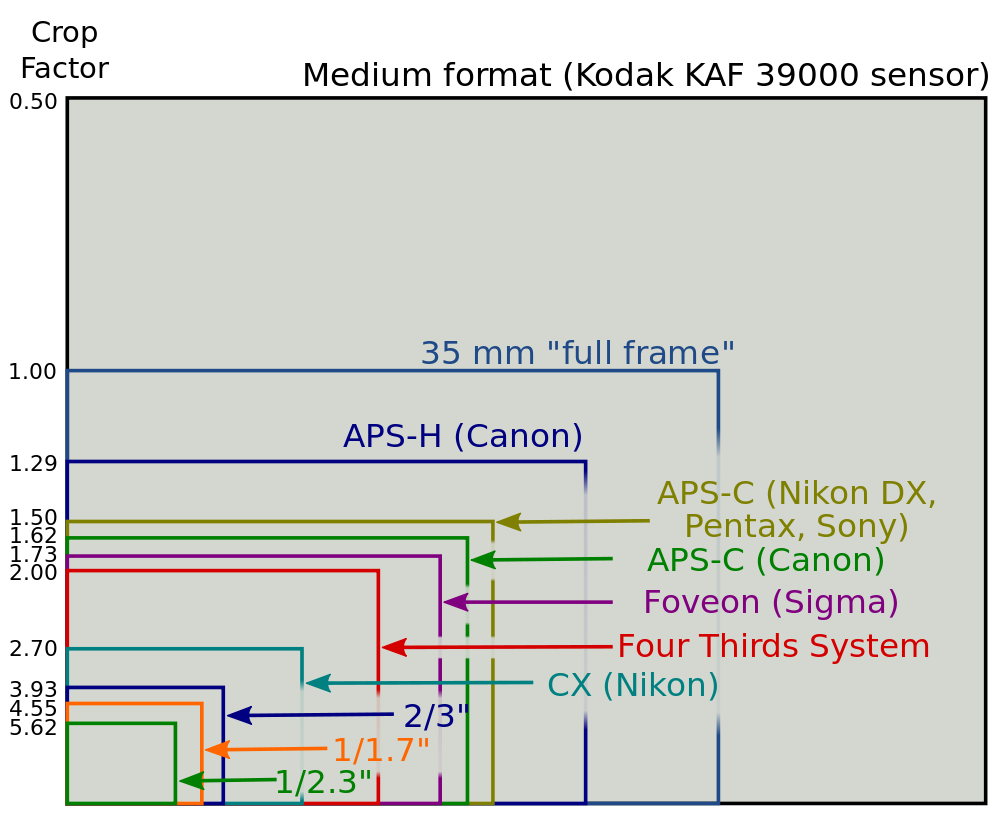
Digital camera sensor format-size names have their roots in television camera tubes. These were measured in inches diagonal, but for various practical reasons, the entire circle isn't used. So, from way back then, there's a concept called "the rule of 16", which says that the usable, actual sensor diagonal for a 1" tube is 16mm. (Yes, it mixes imperial and metric measurements.) So, for each "inch" in a sensor format designation, translate that to approximately 16mm of sensor diagonal. Or, for formats smaller than an inch — very typical, e.g. 1/2.5" — use the corresponding fraction of 16mm.
This rule matches the 1"-format designation for this sensor: 13.2mm × 8.8mm has a diagonal of 15.9mm, and you can see how it roughly applies to the other typical compact digicam formats as well. Usually, there's a little variation and sensor-makers round to the nearest somewhat-standard fraction, but occasionally, as with the 1/1.83" Nokia N8, a very-specific number is given, in which case it's almost certain that they're following the 1" = 16mm rule literally.
More background in this archived article.
Confusingly, 1" does not refer to the size of the sensor, it is instead a "type designation". The 1" refers to a certain size of TV camera tube, of which the usable image area occupied the inner two thirds. The use of such cameras is completely obsolete now but the sizing system remains.
It's arguable whether this is due to doggedly sticking to tradition or an conscious attempt by manufacturers to make it seem like their sensors are larger than they actually are. Probably a bit of both.
For a table of system "types" and their actual physical sizes see
It's a matter of unit conversion/Pythagorean Theorem (or Pythagorean Theorem/unit conversion.) The diagonal measure is (in historical/effective terms) 1 inch. It is similar to purchasing a tv, laptop, screen of any type. The stated size is a diagonal measurement.
