7
\$\begingroup\$

The famous Group f/64, to which Ansel Adams and several other prominent photographers of that day belonged, were named that because of the f-stop:

The term f/64 refers to a small aperture setting on a large format camera, which secures great depth of field, rendering a photograph evenly sharp from foreground to background. Such a small aperture sometimes implies a long exposure and therefore a selection of relatively slow moving or motionless subject matter, such as landscapes and still life, but in the typically bright California light this is less a factor in the subject matter chosen than the sheer size and clumsiness of the cameras, compared to the smaller cameras increasingly used in action and reportage photography in the 1930s.

I presume that was for either a 4×5" or an 8×10" large-format camera. I will presume the latter in my question.

When you capture images on a medium- or smaller format, the magnifications needed to make an 8x10" print increase from the 1:1 contact print of an 8x10" capture. Therefore, just saying something is f/5.6 doesn't tell you the depth of field achieved in the final print.

From Wikipedia, we see this:

size relations

or going the other way, you have this:

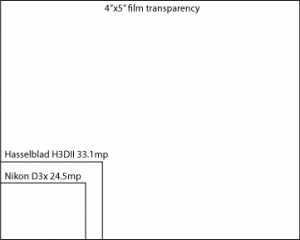
bigger formats

My question is:

If the Group/64 used f/64 for a certain look in their prints with their equipment, what would be the equivalent f-stop in the other format sizes?

  • LF 11×14" — ???
  • LF 8×10" — f/64
  • LF 4×5" — ???
  • Better Light LF Digital — ???
  • MF: 120 film / 6×4.5 cm — ???
  • MF: 120 film / 6×9cm — ???
  • SF: 135 film / 24×36mm / 35mm / full-frame — ???
  • SF: APS-C / 16×24mm — ???
  • Average compact camera — ???
  • Average cellphone camera — ???

Actually, if I just knew the formula based on the diagonal, I could fill out the rest myself. If an 8×10" transparency’s diagonal of 12.8" yields a certain effect stopped down to f/64, I figure we should be able to use a formula of some sort to derive all the others based on their diagonal. I just don’t know what that formula is, and would like to know, please.

I feel like I’ve seen a list like the one I’d like filled out above from Roger Clark, but now I can’t find it.

Improve this question
\$\endgroup\$
2
  • 3
    \$\begingroup\$ Does this answer your question? Can a smaller sensor's "crop factor" be used to calculate the exact increase in depth of field? \$\endgroup\$
    – mattdm
    Commented Jan 10, 2020 at 2:52
  • \$\begingroup\$ The title of your question, as worded, suggests a false assumption that there is a F-stop equivalence. There is no such thing as F-stop equivalence. F-stops are F-stops regardless of the size of the film or the sensor size, pre-determined opening sizes based on doubling and halving, to let a specific amount of light into the camera. Depth of Field based on focal length as effected by F-stops is a different question and should be worded accordingly. \$\endgroup\$
    – Alaska Man
    Commented Jan 11, 2020 at 0:30

3 Answers 3

Reset to default
3
\$\begingroup\$

For depth of field, you can use (for most practical purposes) the ratio of sensor diagonals — the crop factor. See Can a smaller sensor's "crop factor" be used to calculate the exact increase in depth of field?

Exposure per area is the same.

Other factors may also depend on sensor or film size and not translate in this way, of course. A larger area means more light overall with everything else equivalent, and that's an inherent advantage. But it sounds like you're primarily concerned with depth of field, and for that, crop factor will do it.

Improve this answer
\$\endgroup\$
2
  • \$\begingroup\$ What got me thinking about all this was this DOF article and especially the side-by-side comparisons about noise in this article. Even with the same depth of field, you won’t get as many photons in a smaller format, all things being equal, so both depth-of-field and signal-to-noise factor into the image, but differently. Equivalence seems a complicated matter, and not everyone talking about it seems to be talking about the same thing. \$\endgroup\$
    – tchrist
    Commented Dec 25, 2013 at 0:19
  • \$\begingroup\$ Yes, that first article basically says the same thing as my answer but in a lot more detail. :) \$\endgroup\$
    – mattdm
    Commented Dec 25, 2013 at 0:43
3
\$\begingroup\$

Depth of Field (DoF) is always a function of at least these variables:

  • Lens focal length.
  • Aperture expressed as a ratio between the diameter of the entrance pupil (often referred to as the effective aperture) and the lens focal length.
  • the distance from the image plane to the plane of focus.
  • The size of the image plane, often referred to as the camera's format or sensor size.
  • The size of the displayed image.
  • The distance the displayed image is viewed.
  • The visual acuity of the viewer of the image.
  • The resolution (in terms of ppi/dpi) of the displayed image if the smallest unit is within the limits of resolution of the viewer.

As long as the two formats being compared are fairly close to the same height/width ratio then the ratio of the linear measurements of each format can be used to compare DoF when the images from both are displayed at the same viewing size, at the same resolution, and viewed from the same distance by a viewer with the same visual acuity.

Improve this answer
\$\endgroup\$
2
\$\begingroup\$

Depth of field is related to f-stop and focal length, not size of the sensor directly. It is only indirectly related to sensor size because you'd pick a longer lens to get the same scene with a larger sensor. But f/22 on a 300 mm lens will have the same depth of field whether that is used as a telephoto for a "35mm" sensor (actually 24x36 mm), or a mild wide angle on a 8x10 inch large format camera.

Improve this answer
\$\endgroup\$
5
  • 2
    \$\begingroup\$ It is also related to sensor size because you have to magnify an image produced using a smaller sensor by a greater factor to create a print of the same size, such as the standard 8X10 print viewed at 10 inches by a person with 20/20 vision that is the standard applied to most DoF charts. If you change any of those variables (print size/viewing distance/visual acuity of the viewer) the DoF changes for the exact same image file or negative. \$\endgroup\$
    – Michael C
    Commented Dec 25, 2013 at 1:08
  • \$\begingroup\$ @Michael: This depends on how exactly you define depth of field. If it's blur relative to the size of the whole image, then you're right. However, if it's some minimum acceptable blur due to off-focus relative to the sharpness of the lens at focus, then image size doesn't enter into it. Either way, depth of field is a somewhat subjective measure relating to how much degredation you are willing to put up with or that you still consider "in focus". There is no hard sudden edge for off-focus, only what you consider acceptable in that particular circumstance. \$\endgroup\$
    – Olin Lathrop
    Commented Dec 25, 2013 at 17:26
  • \$\begingroup\$ Normally the amount of acceptable blur is defined by the size of the blur circle. With an image from a 35mm size film/sensor printed at 8X10 viewed from 10 inches by a person with 20/20 vision the accepted blur circle is about 0.03mm (30µ). When the blur circle is smaller than 30µ it is perceived as a point at standard size/distance/vision and any blur that size or smaller is considered within the DoF. \$\endgroup\$
    – Michael C
    Commented Dec 26, 2013 at 0:10
  • \$\begingroup\$ But if a negative or digital image is printed at twice the standard 8x10 print (16x20) then the largest blur circles on the negative that will be perceived as single pointsare half the size of the blur circles that will be perceived as points in the 8X10 print, so now the perceived DoF is only the part of the print that comes from the parts of the negative that have blurs circles 15µ or smaller. \$\endgroup\$
    – Michael C
    Commented Dec 26, 2013 at 0:12
  • \$\begingroup\$ See also photo.stackexchange.com/a/34801/15871 \$\endgroup\$
    – Michael C
    Commented Dec 26, 2013 at 0:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.