How rapidly does the focal length-equivalent image change by physically moving the camera?

Question

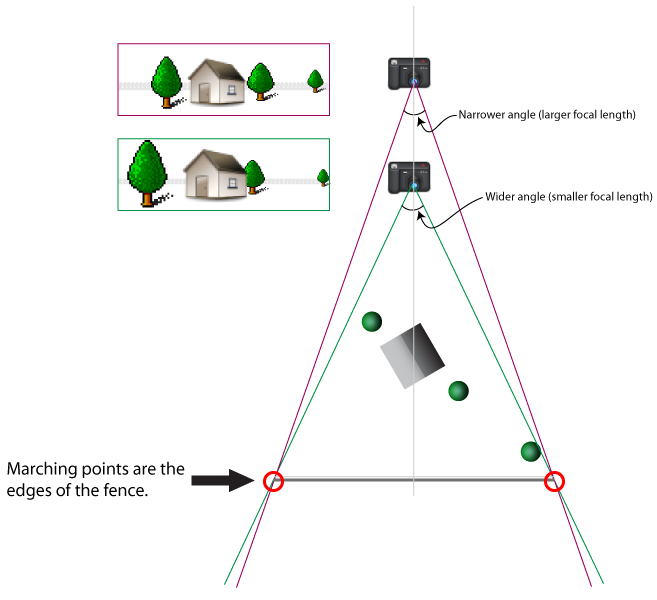
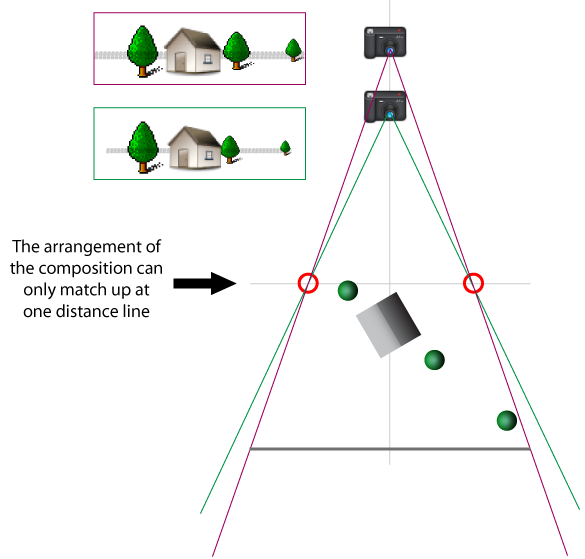
I have recently begun moving from a Nikon APS-C DSLR to the Micro Four Thirds camera while also transitioning from using a viewfinder to using the LCD screen for framing my shots. Because of the size and cost of lenses, I am now shooting with a 14mm (28mm equivalent) lens on the M43 camera instead of the 35mm (52.5mm equivalent) lens on the Nikon. I am getting used to shooting with a wider lens, but I am also curious how my change from viewfinder to screen use effects the actual field of view of the images. Since I no longer hold the camera against my face, the lens is positioned at least 6 inches further forward than it was when using a viewfinder. In other words, rather than going from shooting at 52.5mm to 28mm, I feel like the 28mm lens may behave more like a 35mm lens (relative to my own eyes) due to its different physical position relative to my body.

Do you have any information that can help me better understand the relationship between focal length, field of view, and camera-holding technique?

Answer 1

I have attempted to illustrate mattdm's answer, and maybe expand on a few points.

---

In this first example, two cameras using different focal lengths are photographing the same scene. They have been positioned such that each will capture the full length of the fence behind the house and trees. Notice how because of the different perspective of the wide-angle camera, the second tree appears partly hidden behind the house.

In this second example, the wide-angle camera has been moved to recompose the scene. Now the arrangement of the composition of the house and nearest two trees looks more like what we get from the narrow-angle camera. But notice that by changing our perspective, we are now capturing a wider view of the background than the narrow-angle camera. The fence no longer fills the entire frame.

---

EDIT:

For a more mathematical explanation of the relationship between focal length (field of view), and scene width (subject size), you might want to check out the answers the question Estimating focal length range required for shooting scenario.

Answer 2

The focal length and sensor dimensions form a triangle (or, rather, a pyramid, but horizontally or vertically a triangle), the angle of which is the angle of view. When you move the camera, you're not changing the angle of view, but you are changing the perspective.

That will change the framing, and will be the case that certain different framings line up with the angle of view provided by other focal lengths from different positions — but the perspective is simply a function of where you (or the camera) are located. This is what people mean when they say "you can't zoom with your feet". Changing location inevitably changes perspective in a way that changing focal length does not.