0
\$\begingroup\$

first i am new in the field:

If i have a UAV fly in alt range between 400-1200m, and i want to capture photos like Google Satellite maps, and specifically i want the photos to have the same (or close to) scale of Google maps photos at the most zoomed level (zoom 23-24).

The UAV will have a full-frame mirror-less camera, so i want to know or help me to figure out the required focal lengths.

Thanks.

Improve this question
\$\endgroup\$
3
  • 2
    \$\begingroup\$ If you want your pixels to be the same size on the ground we need to know resolution of the sensor as well. \$\endgroup\$
    – lijat
    Commented Jun 7, 2020 at 20:11
  • \$\begingroup\$ @lijat, The camera will be (most likely) Sony A7R II, so the resolution will be 42.4 MP. \$\endgroup\$
    – abd0991
    Commented Jun 7, 2020 at 20:30
  • \$\begingroup\$ Related question: DSLR, Lens, and other considerations for Aerial Photography \$\endgroup\$
    – scottbb ♦
    Commented Jun 7, 2020 at 20:58

1 Answer 1

Reset to default
3
\$\begingroup\$

For non-wide-angle lenses, and subject distances much larger than the lens's focal length (your use-case certainly applies), the imaging system obeys the following basic equality:

(distance to object, d) / (size of object, O) = (focal length, ƒ) / (size of image, i)

This follows from the relationship of similar triangles as shown below:

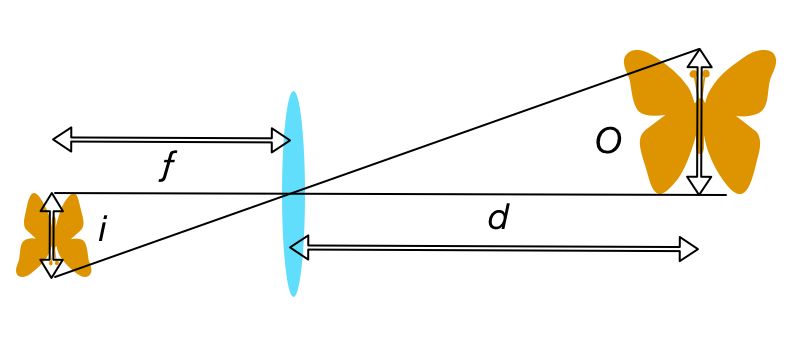
enter image description here

Thus, ƒ = d * i / O. In your case, you want your images to have roughly the same spatial resolution as Google's aerial photos, which have about 6" (15 cm) resolution per pixel. So O = 150 mm. Since that's per-pixel, i is just the pixel size of the sensor you're using. You can look up the actual pixel pitch for your particular sensor, but knowing that a full frame sensor is 36 mm wide, you can just calculate i = 36 mm / (# horizontal pixels, pw).

Putting it together, ƒ = d * 36mm / (pw * O), where d is just your altitude. For example, the Sony a7r II sensor has 7952 horizontal pixels, so at 1,000 m altitude you'd need a lens with focal length ƒ = 1000 m * 36 mm / (7952 * .15 m) = 30 mm.

Lower altitude would give you higher resolution. However, if you wanted to reduce the focal length to maintain a similar spatial resolution, for lenses with a focal length below around 30 mm (on full frame cameras), the lens is considered wide angle. Around 24 mm and lower, depending on the lens, it might not obey the pinhole projection formula (which the ratio of similar triangles describes above). Instead of an equirectangular projection for normal and long focal length lenses, the lens might have projection distortion farther away from the image center. Again, depending on the specific lens you choose.

Improve this answer
\$\endgroup\$
8
  • \$\begingroup\$ First i appreciate ur great explanation. Second, as i said i am beginner so plz bare with me, you said first "For non-wide-angle lenses" and then you got a 30mm as a result which it wide-angle as i know (<35mm), so could u plz explain more this point for me. \$\endgroup\$
    – abd0991
    Commented Jun 8, 2020 at 10:49
  • 1
    \$\begingroup\$ Do note that as one uses wider angle lenses to compensate for nearer distance, the perspective will change. One of the most obvious effects will be that vertical structures at the edge of the image field will appear tilted. \$\endgroup\$
    – Michael C
    Commented Jun 10, 2020 at 15:12
  • 2
    \$\begingroup\$ @abd0991 A 30mm lens can be wide, normal, or long, depending on the size of the sensor. In general, most current schools of thought place the beginning of wide angle for 135 format ("full frame" 36 x 24 mm film or sensors) at around 24-28mm, the normal range from there to about 65mm, and the telephoto range beyond that. For a very small sensor, such as is found in most phones, anything from about 4.8mm to 11mm is considered normal. Wide angle is less than 4.8mm and telephoto would be anythign beyond 11mm. For large format, 200-450mm is considered normal. \$\endgroup\$
    – Michael C
    Commented Jun 10, 2020 at 15:23
  • 1
    \$\begingroup\$ @abd0991 I'm sorry, I made the statement quickly, and then moved on. It probably does require more explanation. Wide angle lenses aren't usually rectilinear. They have bulbous front elements, which are used to capture the wide field of view. This means that the pinhole projection formula (ƒ/ i = d / O) is no longer true. Instead, those lenses use a different projection mapping function. \$\endgroup\$
    – scottbb ♦
    Commented Jun 10, 2020 at 19:05
  • 1
    \$\begingroup\$ @abd0991 So in your case, is you went with a 12mm lens when flying at 400m, you'd have to "de-warp" the image. That would decrease the spatial resolution of the corners of the image. Instead, if you stayed with the 30mm lens when flying at 400m, you'd have 2.5x the spatial resolution than when flying at 1000m. Normally, extra spatial resolution is not bad, and if it were, you could just downsample the image to get back to the 15cm spatial resolution of Google. \$\endgroup\$
    – scottbb ♦
    Commented Jun 10, 2020 at 19:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.