7
\$\begingroup\$

How is a fisheye lens made differently from a normal ortholinear projection lens? How are real lenses acting differently from the ideal "thin lens" or pinhole, in terms of how the 3D scene in front is projected onto the focal plane?

To reiterate, how is a lens shaped differently from the traditional ground lenses in order to do this? "They do" is not an answer!

What does the profile of such a lens look like, and how do sample rays focus on a plane but distort the image? What mathematical concept is it based on?

Improve this question
\$\endgroup\$
1
  • 1
    \$\begingroup\$ This is something I've wondered about recently but have been too lazy to ask. Thanks! \$\endgroup\$
    – JohannesD
    Commented Apr 4, 2016 at 17:39

2 Answers 2

Reset to default
6
+25
\$\begingroup\$

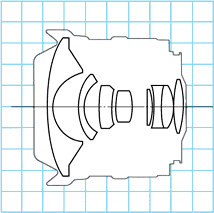
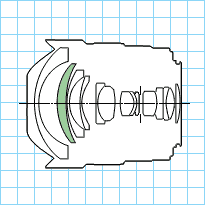
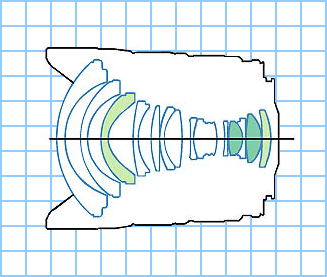
The assumption that aspheric lenses create "abnormal" projections is incorrect.

There is nothing "normal" in normal spherical lenses, except that we can produce them cheaply. You don't get rectilinear projection just because you used spherical lenses, you have to struggle for accurate rectilinear projection (if that's your goal). Pinhole is automatically rectilinear, that's simply geometry, but once we depart from thin lenses, there are many mutually influencing and nonlinear parameters and many degrees of freedom. Change lens curvature, spacing, index of refraction - and you get different aberrations, projections, distortions, everything. Apsherical lenses is only one step further, not something fundamentally different.

By the way, this is Nikon US Patent 3,737,214 - fisheye lens consisting of only spherical lenses.

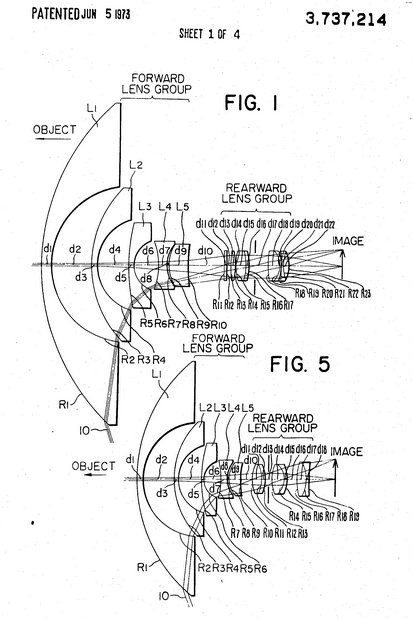
Nikon patent

How can you design such thing? The basic ideas come from great predecessors and then, nowadays you use computer application that calculates everything and can automatically optimize certain parameters following the given criteria. For example this is from OSLO (commercial, free trial available):

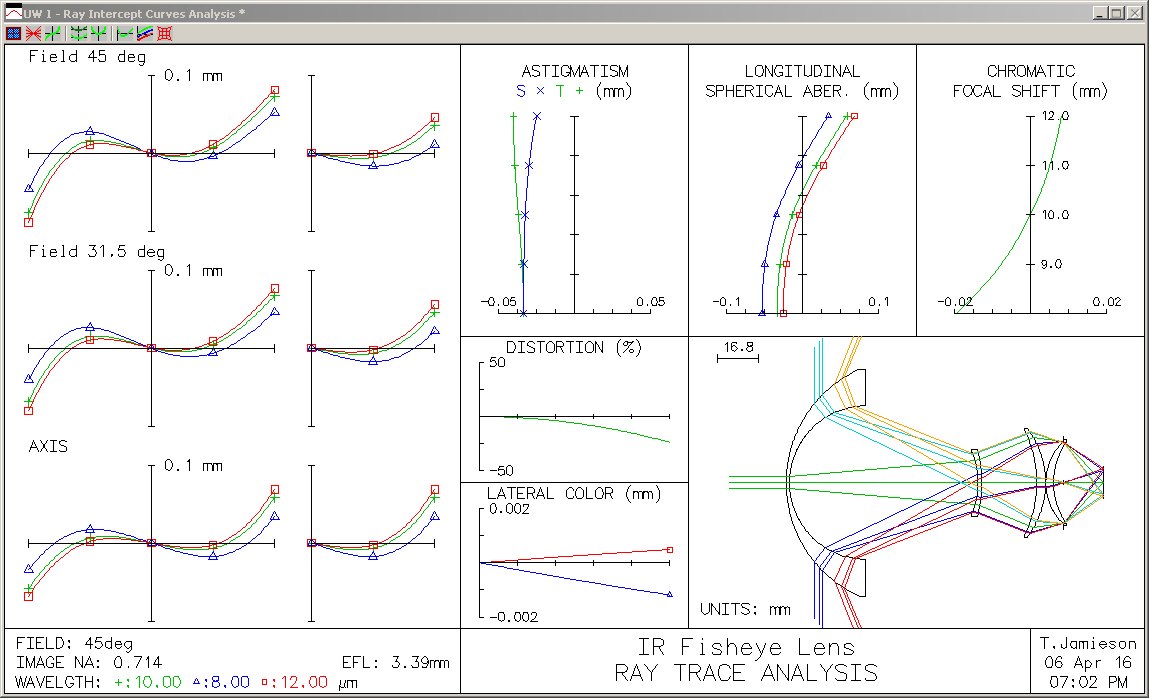
OSLO Example

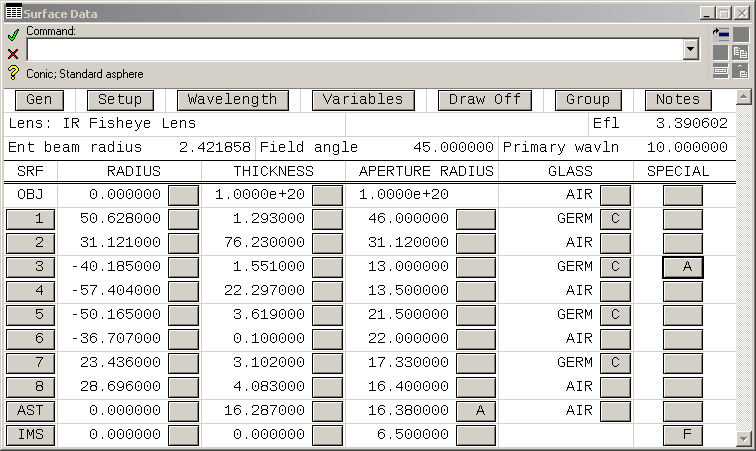
OSLO Spreadsheet

Playing with such software is great way to feel how the lens design really works (even if you only use it for "breaking" existing examples and watching how the performance deteriorates)

Improve this answer
\$\endgroup\$
3
  • \$\begingroup\$ "Many degrees of freedom": so, a single lens like L1 might not focus to a plane at all, on its own? \$\endgroup\$
    – JDługosz
    Commented Apr 6, 2016 at 17:28
  • \$\begingroup\$ Yes, and one of the basic aberrations known in optics is "spherical aberration" - called so because it's the most pronounced defect of a single spherical lens. By the way, L1 is diverging so it can't focus but creates virtual image instead. \$\endgroup\$
    – szulat
    Commented Apr 6, 2016 at 23:50
  • \$\begingroup\$ @szulat: Informative and concise, with excellent references. Thank you! \$\endgroup\$
    – HamishKL
    Commented Apr 7, 2016 at 8:33
0
\$\begingroup\$

By the shape, density, and refractive index of each lens element as well as the physical relationship between each element to the others in the lens. In short, it depends on the lens' design.

Here is a Canon EF 15mm f/2.8 fisheye (left), an EF 14mm f/2.8 L (right), and an EF 14mm f/2.8 L II (bottom) block diagram:
14mm fisheye 14mm rectilinear 14mm II
Notice the difference in the shape of the fisheye's front element compared to the similar shape of the two rectilinear lenses? That's what makes the projection of the lens a fisheye shape, rather than a rectilinear shape.

The fisheye lens had a flatter surface on the front side of the front element but a much narrower radius curve on the back side. This results in a lens element that is much thicker on the edges than in the middle and is what allows the projection to be shaped the way that it is. The rectilinear lenses (with the same focal length as the fisheye) have front elements that are more curved on the front, but the curve of the back of the lens has a radius much closer to the radius of the curve of the front of the lens and are only moderately thicker at the edges vs. the center of the lens when compared to the fisheye. Everything behind the first two elements of the fisheye lens, and behind the first four elements of the other two lenses are pretty much either focusing elements or corrective elements that don't have much influence in the shape of the projection of the lens - that has been determined at the front end.

Improve this answer
\$\endgroup\$
7
  • \$\begingroup\$ It may be worth noting that the correction itself is achieved using asperical elements (not drawn obviously on the schemes). I did not see the legend for those schemes but it seems that light green elements are aspherical. \$\endgroup\$
    – Euri Pinhollow
    Commented Apr 4, 2016 at 12:08
  • 1
    \$\begingroup\$ But how does a lens produce a different mapping function, at all? No matter what focal length, or which sides are curved to acheive that, the projection is the same as that of a pinhole. \$\endgroup\$
    – JDługosz
    Commented Apr 4, 2016 at 13:55
  • 4
    \$\begingroup\$ "Because they are made that way" is not an answer. "Because one of the elements can do that" just pushes the question to apply to that element. Ok, how is a single-element simple lens made to produce a (say) Equisolid angle mapping as opposed to the Gnomonical that you get if the lens "just focuses"? \$\endgroup\$
    – JDługosz
    Commented Apr 4, 2016 at 14:00
  • 2
    \$\begingroup\$ @PinhollowEuri The aspherical elements correct for CA, field curvature, and small amounts of pincushion or barrel type distortion. They have little influence on the shape of the lens' projection at the scale of the difference between a fisheye or rectilinear projection. The shape of the objectives on the front do that. \$\endgroup\$
    – Michael C
    Commented Apr 5, 2016 at 7:08
  • 1
    \$\begingroup\$ @JDługosz I guess if you wanted to argue semantics, pretty much every element in modern lenses is aspherical. But when a manufacturer touts a lens with an aspherical element or two or three, they are generally referring to the corrective elements for CA, field curvature, and the like that use materials with a refractive index that is either very high or very low when compared to typical optical glass. \$\endgroup\$
    – Michael C
    Commented Apr 6, 2016 at 5:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.