1
\$\begingroup\$

Clearly, a lens with focal length F and aperture 1/f has to be at least F/f mm wide. Maybe a little more to accommodate the angle of view.

However, lenses tend to have wider front elements than F/f. For example, my Sony 24-240 3.5-6.3 has filter thread 72 mm, and the front element is not much smaller than that. But, 240/6.3=39, so it would seem that, theoretically speaking, the front element shouldn't have to be much wider than 39 mm, if one would account only for F/f.

Is there another physical reason for that?

Improve this question
\$\endgroup\$
0

1 Answer 1

Reset to default
5
\$\begingroup\$

Lenses with very narrow angles of view require front elements that are roughly equivalent to the size of the entrance pupil. A typical telephoto prime lens will have a front element less than 10% larger than the entrance pupil at the lens' maximum aperture. This is because the light rays collected by the lens are almost perpendicular to the imaging plane and the entrance pupil will not be much larger than the diameter of the front element.

But with wider angles of view and their closer subject distances, the entrance pupil can be much larger than the front element:

A simple single element lens:

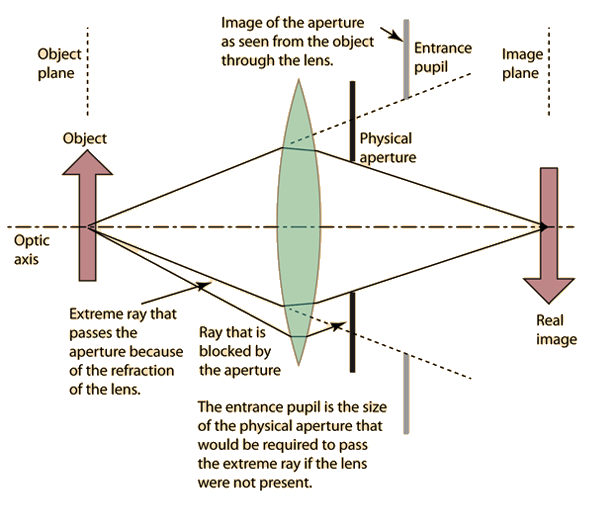
enter image description here

A multiple element compound lens:

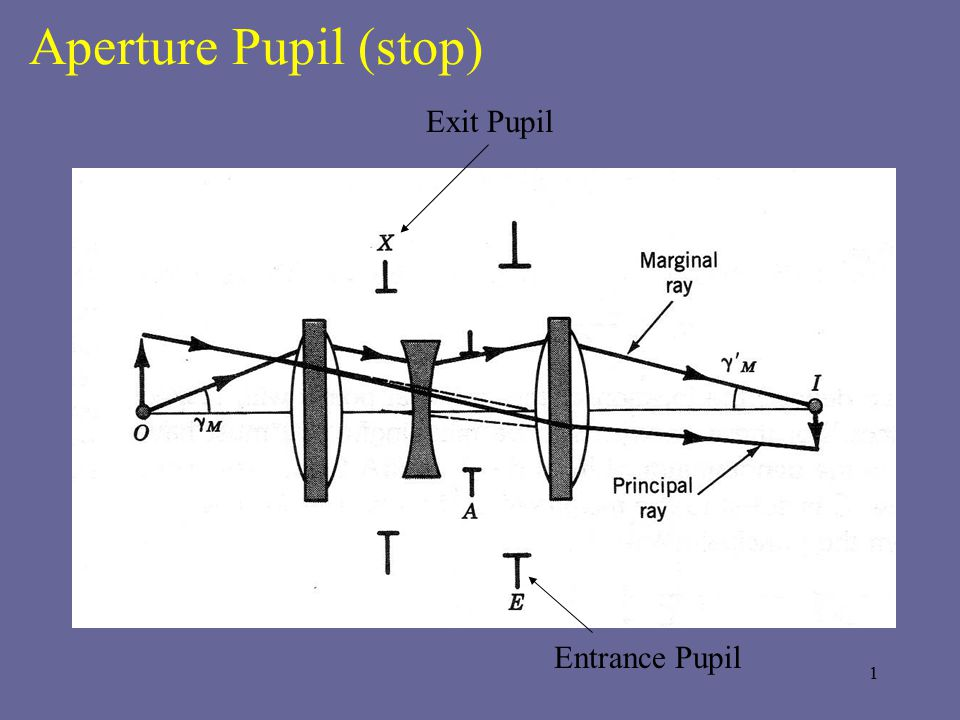
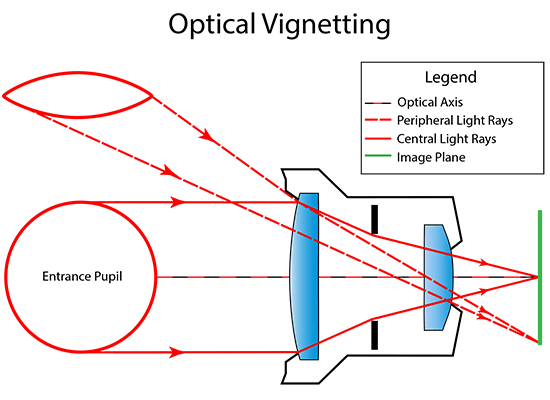
enter image description here

If the front element of a wider angle lens were only large enough for the entrance pupil to be fully visible from subjects centered on the lens' optical axis, the lens would severely vignette the light coming from the off axis portions of the frame. Thus wide angle lenses tend to have much larger front elements than the size of the entrance pupil so that a larger portion of the entrance pupil is visible from the more peripheral parts of the field of view.

When portions of the lens' field of view are obstructed from a full view of the entrance pupil, it can result in dark corners and oddly shaped out of focus highlights. Consider wide aperture lenses with even a normal field of view:

enter image description here

Such a lens is said to have "cat's eye" bokeh:

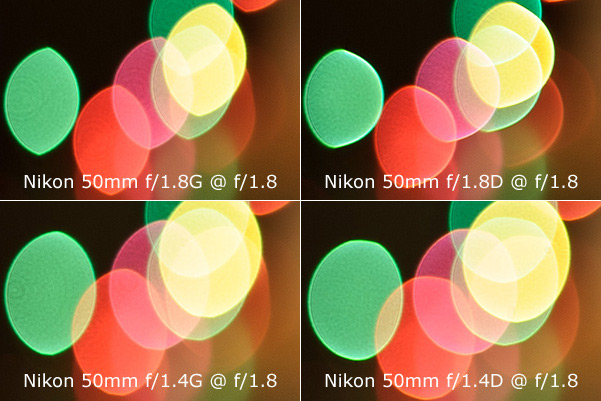
enter image description here

Even when there is no mechanical vignetting caused by the lens barrel, from wider angles the entrance pupil appears to be an oblong shape, rather than a circle.

enter image description here

Compare these examples, all intended for full frame cameras:

  • Canon EF 300mm f/4 has 77mm filter threads. 300mm/4 is 75mm
  • Canon EF 100mm f/2 has 58mm filter threads. 100mm/2 is 50mm
  • Canon EF 85mm f/1.8 has 58mm filter threads. 85/1.8 is 47mm
  • Canon EF 50mm f/1.4 has 58mm filter threads. 50mm/1.4 is 36mm
  • Canon EF 35mm f/2 has 67mm filter threads. 35/2 is 17 mm
  • Canon EF 24mm f/1.4 has 77mm filter threads. 24mm/1.4 is 17mm

Your 24-240mm f/3.5-6.3 lens having a near 72mm wide front element probably is more about reducing vignetting at 24mm and f/3.5 than it is about the needed entrance pupil for 240mm and f/6.3.

Improve this answer
\$\endgroup\$
14
  • \$\begingroup\$ Ahhh, "cat's eye bokeh" is what it's called. Also very prominent on the Canon 50mm f1.4 SSC IIRC... \$\endgroup\$
    – rackandboneman
    Commented Jan 26, 2019 at 22:16
  • 1
    \$\begingroup\$ Maybe it's just me, but I'm still trying to figure out how "the entrance pupil can be much larger than the front element". It can (and usually is) certainly be much larger than the actual physical diaphragm, but a virtual image that goes outside the physical boundary of the front element...? \$\endgroup\$
    – twalberg
    Commented Mar 23, 2019 at 13:12
  • \$\begingroup\$ @twalberg What is it about the first diagram in the answer above that is not self evident? This diagram shows two additional dotted lines that show why the entrance pupil is the distance it is behind the lens. The entrance pupil is viewed through the front of the lens from a point on the lens' optical axis at the focus distance, but the aperture opening appears to be a certain distance behind the front element, not on its surface. \$\endgroup\$
    – Michael C
    Commented Mar 23, 2019 at 17:52
  • \$\begingroup\$ This is why wide angle lenses often (almost always) have much larger elements that required by the size of their e.p. - to prevent vignetting because the entire e.p. would not be visible from the edges of the lens' maximum AoV. But they don't have to be that large if light fall off on the edges is not a concern. \$\endgroup\$
    – Michael C
    Commented Mar 23, 2019 at 18:05
  • \$\begingroup\$ @MichaelC That doesn't answer my question. How can an entrance pupil, which is admittedly a virtual image, appear larger than the physical limits of the front lens element? Viewing from on-axis at any point, before, at or behind the focus distance, the entrance pupil must fit within the confines of the front element, otherwise light must be required to pass through the opaque portions of the lens outside the physical boundaries of the front element. \$\endgroup\$
    – twalberg
    Commented Mar 23, 2019 at 18:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.