As your lens's focal length gets longer, fewer photons pass through the lens to hit the mirror/sensor.
Why don't you see darkening when you look into the viewfinder and zoom in with a zoom lens, and brightening vice versa?
Why don't telephoto lenses need longer shutter times than wide-angle lenses?
The answer to this question revolves around explaining how zoom lenses function because you are correct in your observation: As you zoom to higher and higher magnifications the image dims unless somehow compensation is applied. Suppose you zoom from 25mm to 50mm, should the working diameter of the aperture remain unchanged, image brightness would suffer a 4x loss as to its intensity. Stated differently, each doubling of the focal length will dim, it will be just 25% as bright as it was before the zoom. If true, how is this light loss prevented?
The amount of light energy that can enter the lens is directly related to the working diameter of the iris diaphragm (aperture). The larger the working diameter the more surface area, the more light the lens can gather.
The modern zoom lens has a trick up its sleeve that keeps the image brightness the same thorough most of the zoom. Some high end zooms keep the image brightness throughout the zoom. How this works: The diameter of the aperture as seen when looking into the lens from the front appears larger than it actually is. This is because the front group of lens elements of the zoom lens magnifies thus the diameter of this entrance circle appears larger than reality.
Further, as you zoom, the distance from the front lens group and the iris diaphragm also change. This induces an apparent diameter change. The fact that it is apparent and not a real change is unimportant. From the outside looking in, this change appears real and this action allows more and more light energy to enter as you zoom.
As I said earlier, some high end zooms are good to go through the entire zoom. These are called constant aperture zooms. Lower priced zooms keep a constant aperture until the last 80% or so of the zoom, these fail and suffer the light loss you are asking about.
Why doesn't the picture become darker the more you zoom in?
If the entrance pupil size remains constant, it does.
But very few zoom lenses, even those with variable maximum apertures, maintain the same entrance pupil size as the lens is zoomed.
As your lens's focal length gets longer, fewer photons pass through the lens to hit the mirror/sensor.
Again, only if the entrance pupil size remains constant.
But to maintain the same f-number, the diameter of the entrance pupil is required to scale up at the same rate as the focal length. If you double the focal length you must also double the diameter of the entrance pupil, which quadruples the area of the e.p., to maintain the same f-number.
The physical size of the diaphragm is only part of what determines the maximum aperture, expressed as an f-number, of a lens. Magnification between the front of the lens and the location of the diaphragm also plays a part. The f-number of an aperture is determined by the ratio of the lens' focal length divided by the diameter of the entrance pupil, often referred to as the effective aperture.
In simple language, the entrance pupil diameter is defined by how wide the opening of the diaphragm appears when viewed through the front of the lens.
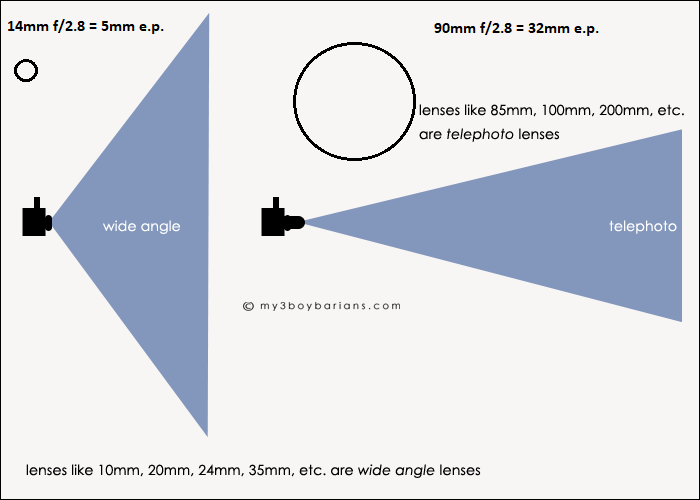
In your example, a 14mm lens with a 114° angle of view has a 5mm wide entrance pupil at f/2.8. For DSLRs and even most mirrorless cameras, a 14mm lens is what is called a retrofocus design. It's more or less the equivalent of a telephoto lens turned around backwards. So the 'magnification' between the aperture diaphragm and the front of the lens is negative. That is, the entrance pupil appears smaller than the actual size of the physical diaphragm! On the other hand, a 90mm lens with a 27° angle of view requires an entrance pupil 32mm in diameter for f/2.8. That's 6.4X wider, or 41X more area than the 5mm entrance pupil of the 14mm lens at f/2.8.
When constant aperture zoom lenses are moved to change the focal length, the magnification between the front of the lens and the diaphragm is what normally changes, not the physical size of the diaphragm. This change in magnification is what allows the entrance pupil to appear larger at longer focal lengths and smaller at shorter focal lengths for the same physical diaphragm. A 70-200mm f/2.8 lens has an entrance pupil 25mm in diameter at 70mm and f/2.8. At 200mm the entrance pupil at f/2.8 is a tad over 71mm wide. The actual physical diaphragm is the same size in both cases. What has changed is the amount of magnification between the diaphragm assembly and the front of the lens.
Note that this same principle is usually in play with variable aperture zoom lenses as well. Take, for example, an 18-300mm f/3.5-5.6 zoom lens. At 18mm the entrance pupil for f/3.5 is roughly 5.14mm wide. At 300mm the entrance pupil for f/5.6 is over ten times that at 53.6mm wide. Notice that most zoom lenses that max out at 300mm and f/5.6 have front elements that are slightly larger than 54mm in diameter. The needed entrance pupil size is the reason! If the entrance pupil at 300mm were still 5.14mm wide as it is at 18mm and f/3.5, the maximum aperture at 300mm would be f/58!
So why don't all zoom lenses use enough magnification to remain at constant aperture throughout the entire zoom range? Primarily the cost associated with the additional size, weight, and complexity needed to produce a constant aperture lens.
The f/stop system of numbering is specially invented to ensure that different lenses at the same f/stop number will see the same exposure. This includes your wide angle and telephoto lenses. F/stop number = focal length / effective aperture diameter.
Also, the wide angle lens can collect more overall total of photons (from a wider area). However a focal length 2x longer (100 mm vs 50 mm) does make the subject appear 2x larger, except our telephoto lens (and same sensor size) crops our view into 1/4 the area still visible. Assuming our subject was a large evenly lighted blank wall (no special areas to complicate this), then we see 1/4 the light (photons, your argument), but in 1/4 the area, which is the same light per unit of area. Exposure is about light per unit of area, Not about total photons in the whole frame area (a bright right hand edge of frame adds photons, but does not change the proper exposure of a dark left hand side).
Your pupils dilate to compensate while looking through the viewfinder.
Yes, your reasoning is correct, the picture becomes darker as you zoom in, assuming all other factors stay unchanged.
When the automatic exposure mode is used, your camera simply compensates for the darkening by adjusting exposure time, ISO, or aperture. Switch to manual mode or examine the displayed photo settings while zooming to see the relationships between those parameters and the apparent brightness.
