Is there a website / app that will tell you approximately how much distance a person should move so that they are able to frame the same shot at different focal lengths?
Say for example, I'm using an 85mm lens to focus on a person head to toe. I then change the lens to a 135mm lense, how much further should I step back, so that I'm able to focus on the same person head to toe?
I do realize that the pictures from the 85mm/135mm would not look the same but I am ok with that.
It's actually far simpler than any of the answers posted so far! You don't need trigonometry, or field of view calculators at all, all you need is multiplication and division!
Firstly (all else being equal) the size of your object in the image is directly proportional to the focal length (if you double the focal length you double the size).
So if you know the subject distance for your 85mm lens, you can work out the subject distance for a 135mm lens as follows:
new subject distance = (135/85) x old subject distance
For the second case (known subject height), we can exploit the fact that the triangle formed between object and the lens aperture is similar to the triangle formed between the lens aperture and sensor. Thus the laws of similar triangles can be used to find the missing side (which represents the subject distance). In other words the size of your subject on the sensor divided by the focal length is the same as the size in real life divided by the subject-camera distance.
So if you know the subject height and the sensor height (between 15mm and 16mm for most crop sensor DSLRs in landscape orientation) then you can work out the correct distance as follows:
distance = (real height x focal length) / sensor height
All units must match, so if you use the focal length in millimetres, then the subject height, sensor height and distance must all be millimetres.
TLDR: No because you need an additional variable, either the height of what the subject is that fills the frame or the distance at which you are focused (infinity doesn't work though).
Long answer...
To do this you can use simple trigonometry, but you would need to know either the current or desired distance to subject or the size of your subject (assuming that the size of your subject fits perfectly inside of your frame: feet at the bottom, head at the top and no room on either side). Because focus is obtained for objects at a certain distance, if your lens has a focus window then you can estimate your distance based on that. This also assumes your lens is aimed perpendicular to your subject, which if not will skew the results (though probably not perceptibly).
Make sure your calculator is set to degrees and not radians. I used lenshero.com to get the angle of view for the different lenses.
If I'm right this is an awesome example of how to use trig in real life. If I'm wrong we'll just blame it on public schools.
If your camera tells you at what distance you are focused this becomes much easier because you know a and d and can calculate for h, but you won't have to worry about the focal plane being parallel with the subject because it's automatic (it's an imaginary subject that may be different from the actual subject, but when we are solving for d we need the actual subject to be parallel to the focal plane to make the math work right).
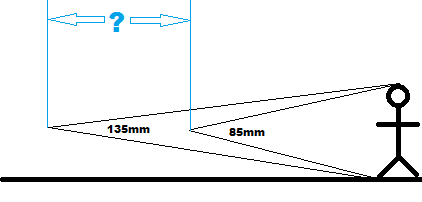
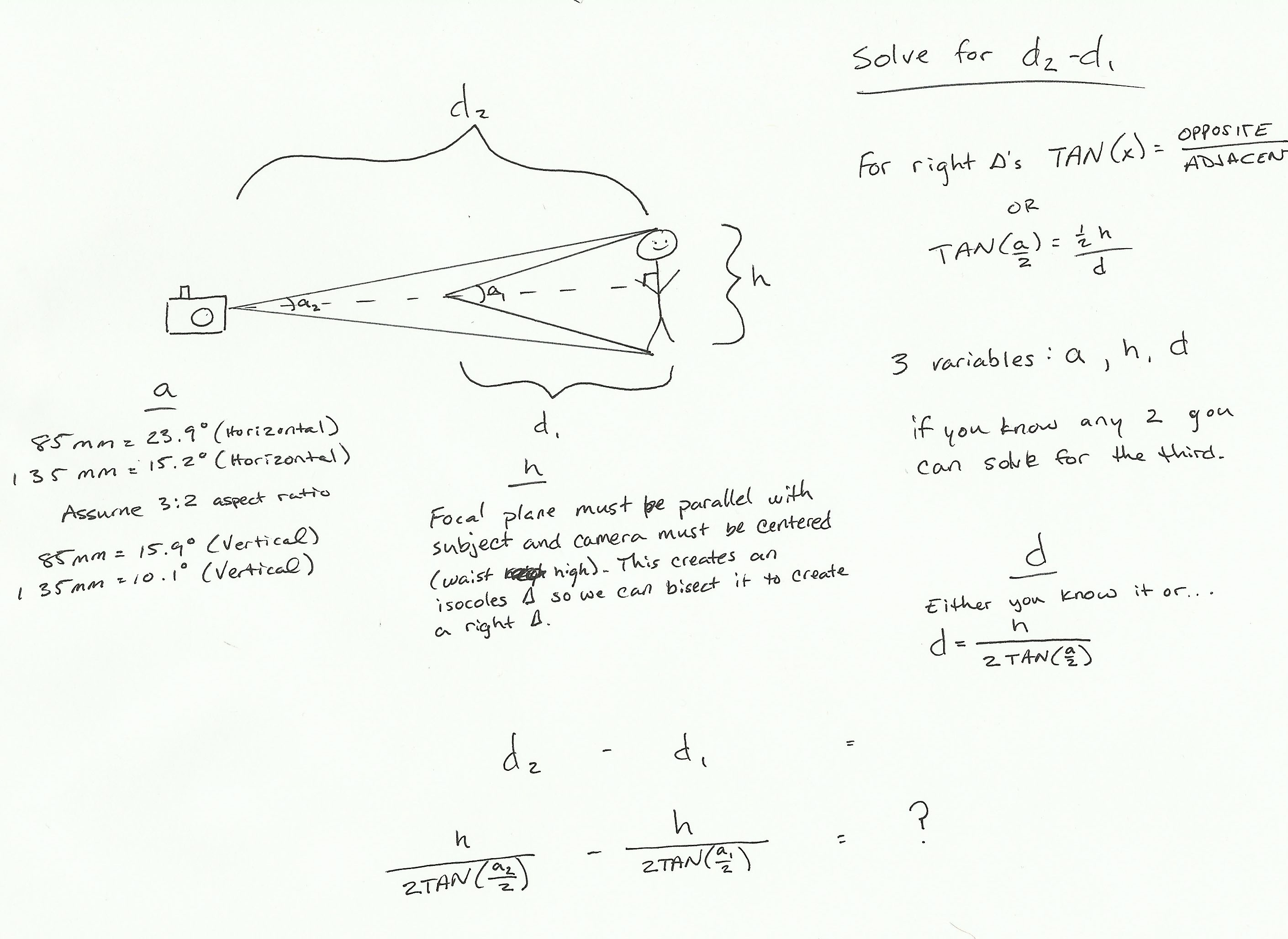
What @tenmiles says is correct. I would make two modifications to make this more useful (which is what I played with before). Note: you have to look up the angle of view (various web sites or the manufacturers' sites).
First, since you want the same "view" from both lens, you are indicating the distance (d2) where h is the same as it is with the other lens. For that part, instead of using h/2, you can just use "w", implying 1/2 of the width of angle of view for a spscific distance.
Second, I'd solve the equation, so that it is in terms of d1 and d2. So...
w/d1 = tan(angle1/2), and w/d2 = tan(angle2/2)
which gives:
w = d2 * tan(angle2/2) and w = d1 * tan(angle1/2)
These are equal, so:
d2 * tan(angle2/2) = d1 * tan(angle1/2)
In other words:
d2 = d1 * tan(angle1/2) / tan(angle2/2)
You can calculate (once), the tangents, so that you have d2 in terms of some constant times d1. Then, you can use a spreadsheet and plug in d1 and see what d2 comes out to. I did this for two lenses I had and found that what I'd shoot at 10' with one, I'd need to be at 14' for the other, etc.
