does focal length change across an image?

Question

If an image is taken of a flat surface parallel to a (presumed perfect) lens, is the scale of objects (and therefore focal length) uniform across the whole image? eg. Would an object in the centre of an image measure smaller than on an edge?

Answer 1

There's really no such thing as a 'theoretically perfect' rectilinear lens with a perfectly flat field of focus and no geometric distortion. Even a lens that perfectly matches its blueprint would not demonstrate such perfection. A theoretical thin lens demonstrates field curvature as well as several other aberrations. Various corrective elements used to correct these aberrations result in a field of focus that is shaped more like a lasagna noodle than a flat plane. In a very high quality flat-field lens the lasagna noodle is almost flat, but it still has some waves in it. Compound lenses also have geometric distortion.

To put it another way, geometric distortion is not caused by our inability to manufacture a lens that perfectly matches its blueprint. The distortion is inherent in the design of the lens itself and the properties of refractive optics and visible light. There is no theoretical way to perfectly correct for all geometric distortions at all wavelengths of visible light simultaneously. This is because the same refractive lenses will bend different wavelengths of visible light by slightly different amounts. If we make a lens perfect for one wavelength, wavelengths longer and shorter than the optimal wavelength will still demonstrate aberrations such as chromatic aberrations, which is a difference in magnification due to differences in wavelengths, for that lens design. The slight difference in magnification for different wavelengths of visible light through the same lens elements is what creates many of the classic lens aberrations that decrease the 'sharpness' of a lens.

Geometric distortion is corrected to a greater or lesser degree, depending on the particular design of the lens. Lens distortion results in items in parts of the lens' field of view being magnified more or less than in other parts of the field of view. If the center is magnified more than the surrounding edges and corners, then we call that barrel distortion because it looks like the side of an old wooden barrel that bulges out in the center. If the center is magnified less than the edges then we call that pincushion distortion because it resembles a pincushion used by seamstresses. There are cases where there is a mixture of both types of distortion and we usually call that mustache distortion.

Although we don't usually refer to the various magnifications in different areas of a lens' field of view due to geometric distortion as having different focal lengths, that is what is occurring and it can be measured.

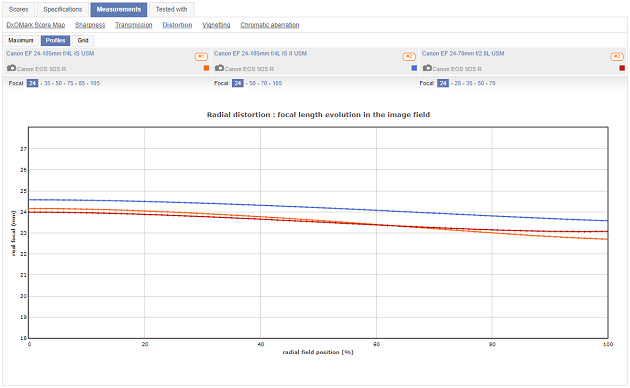
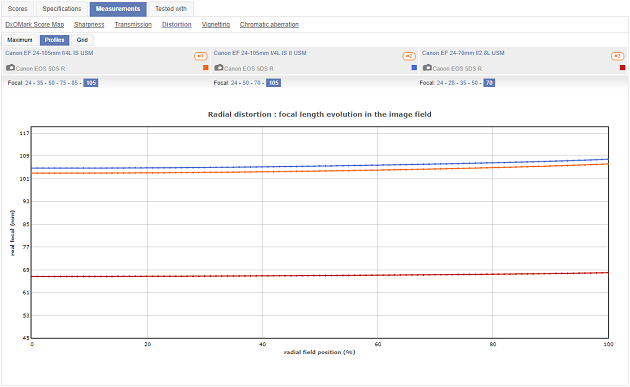
DxO Mark demonstrates this with their "Distortion Profile" chart. The vertical axis is 'real focal length' in millimeters. The horizontal axis is position from the center of the lens' field of view on the left to the edge on the right. These three lenses demonstrate barrel distortion when the lenses are zoomed all the way out to 24mm. Notice that the center of two of the three lenses have a magnification of right at 24mm, but the magnification drops to around 23mm by the edges. The other lens starts in the middle at 24.5mm and drops to about 23.5mm at the edge.

At the other end of their focal length ranges, these three lenses show pincushion distortion, which is fairly typical of zoom lenses. Notice that the 24-70 lens only increases from about 67mm to about 68.5mm from center to edge. The two 24-105 lenses each increase a bit more, from around 105mm to 108mm and from 103mm to about 107mm.

In general, rectilinear zoom lenses typically demonstrate more geometric distortion than rectilinear prime lenses do, particularly at the extremes of their focal length ranges. Even most very expensive zoom lenses demonstrate more geometric distortion than many well designed and more modestly priced prime lenses. The larger the ratio between the shortest and longest focal length of the zoom lens, the harder it is to control distortion at both ends of the focal length range. Correcting it more on one end of the focal length range tends to make it worse on the other. This is particularly true of lenses that have retrofocus designs on the wide end of their focal length range and transition to telephoto designs by their longer focal lengths. This, along with the narrower maximum apertures, is one of the biggest disadvantages of very large ratio 'all-in-one' zoom lenses such as an 18-300mm lens.

Answer 2

There are "barrel" and "pincushion" distortion optical aberrations which involve variation in magnification across the image -- this isn't discussed in terms of a change in focal length.

With barrel distortion, objects closer to the center of the image are magnified more than the sides. So instead of looking like a flat plane you see something that looks like the side of a barrel. With pincushion distortion you get more of a concave effect.

For more information, see Wikipedia's article on Optical aberration

Answer 3

A rectilinear lens is a lens that keeps straight lines straight. It is easy to see that this means that objects of the same size remain so no matter where in the image they lie.

The typical lenses we use are rectilinear (barring optical errors, but you supposed a perfect lens), but very wide angle lenses usually are curvilinear fisheye lenses, which do not keep lines straight and sizes constant.

Answer 4

By definition, focal length is a measurement taken when the lens is imaging a far distant object (∞ infinity). When imaging nearby objects, the distance lens to image is elongated. We are now talking, “back focus”.

All lenses project a circular image that is far larger than the digital sensor or film. This image is at its brightest and sharpest in the central area of the projected image. Both brightness and sharpness falls off at the edges of the frame. That portion of the image that is pictorially useful is called the circle of good definition. The reasons for the falloff are many however, looking back at the lens from the center, one sees a circle, looking back from the edges of the frame, one sees an oval. In other words, the circular aperture is perceived as an oval when viewed from the edges of the format. You need to know that the surface area of the lens is abridged as it projects an image near the boundaries of the circle of good definition. Here less light arrives plus the circles of confusion are also oval in shape. This is alike the circular beam of a flashlight shining on a surfaces from an angle. This falloff is called cosign error and accounts for less light energy arriving at the edges (vignette). Because of these factors and others, definition falls off at the edges.

Another aspect that comes into play is depth of focus, a counterpart of depth of field. We are talking about the tolerance distance image to lens. Converging rays coming from the center come to a focus at a different distance as compared to rays coming from the margins of the lens. Thus the back focus distance varies across the image expanse.

The bottom line is, all lenses suffer from aberrations that must be mitigated. Which one will be the most highly corrected is based on the design task of the lens. Variations in back focus distance from center to edge is one of the maladies we are forced to deal with.