Can the diffraction limit be overcome with superresolution?

Question

As the title says, can the diffraction limit be overcome with superresolution techniques? Or is this the absolute hard limit in optical photography without making special assumptions? If this is the hard limit, what is an illustration of why this is the case?

"Without making special assumptions" in this case means the techniques of superresolution microscopy - structured light, laser beams etc.

To illustrate the point: Can superresolution beyond the diffraction limit be achieved by taking multiple exposures from slightly different angles and positions and feeding them into [SR approach here]? Even with the added assumption of a diffraction-limited system (High resolution camera and lens)?

UPDATE Thank you for your answers. However it feels to me more like you explained to me what diffraction means rather than if it is possible to overcome the diffraction limit under reasonable assumptions. To clarify further: In a relatively controlled environment, where you can expect the subject to be stationary and the lens/aperture diffraction to be the limiting factor of resolution (as opposed to sensor resolution), do techniques exist to increase detail beyond this diffraction limit without the aforementioned "special assumptions"?

Answer 1

Can the diffraction limit be overcome with superresolution techniques?

Sort of, to a limited degree. Using sub-pixel shifting of the imaging sensor, in effect you are increasing each pixel size while keeping their spacing the same. Of course, it is not physically possible to build sensors where individual pixels are larger than their pitch (center-to-center spacing). But mathematically, this is basically what's happening.

That sounds great, but how does that overcome diffraction limits?

As Michael Clark stated in his answer, a camera system is diffraction limited when the size of the Airy disk (the blur) caused by diffraction becomes larger than the size of a digital camera's sensor pixels.

The size and nature of the Airy disk is not something you can overcome — it's a function of the wave-like behavior of light, the aperture size (usually assumed to be circular), and the wavelength of the particular light in question).

But if you can increase the size of the pixels while still packing the same number of pixels in the same area, you can "push back" the diffraction limit a bit farther. And that's what sub-pixel shifting of the image sensor does.

So it's not overcoming the diffraction limit per se, it's more like moving the goalposts a little bit.

The upper limit of sub-pixel shifting superresolution is an apparent twofold increase in resolution.

You don't get something for nothing. What's the tradeoff?

Well, as you mentioned, it requires a non-moving subject, that's one of the limits of applicability. As John stated in his answer, you are using the temporal-based certainty (i.e., there is no motion in the scene, so it exists independent of time) to take multiple images (which takes time, but who cares, you have plenty of it when the subject isn't moving) that help you increase your spatial information / knowledge about the scene.

Answer 2

In essence, no - the diffraction limit cannot be overcome if we want to create a natural color picture.

Even with an optically perfect lens, the resolution will always be limited by the Rayleigh Criterion as pointed out by the other answers (if no other limits like e.g. lens imperfections lower our resolution further).

Common super-resolution techniques that can be used in this setting today try to increase the resolution by recovering the original image that we can only sub-sample with our limited sensor resolution (combining multiple slightly offset images) and/or modelling the imperfections of the optical system that prohibit it from reaching the diffraction limit. Another concept worth mentioning here is apodization, but this does not overcome the diffraction limit but merely removes the non-central maxima of the Airy disk.

The resolution limits are extensively studied in microscopy, so it is useful to look at the techniques developed there. However, all the techniques that provide resolution beyond the diffraction limit are not applicable to form a natural color image and without altering the subject.

Example

For an Airy disk that results from a given aperture we can take a look at two extreme cases. In the first case we have a sensor that has a significantly higher pixel pitch than the size of the Airy disk. In this case, we severely subsample the Airy disk and only know that there is a blob of light hitting a single pixel, but nothing about its shape.

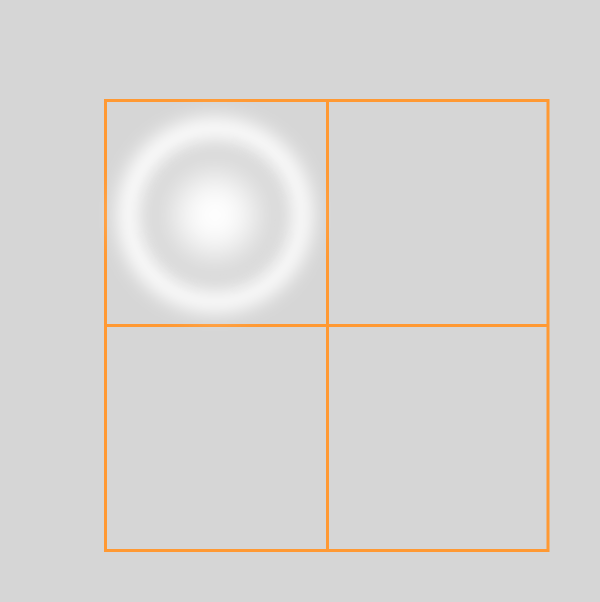
But we can get more information on the shape if we create multiple exposures that are ever-so-slightly shifted, effectively creating virtual pixels of a smaller size when we apply our math appropriately.

(Sidenote: With today's fill factors of close to 100%, the achievable resolution increase is limited. This is because we are sampling with a rectangular window instead of a single point, but I couldn't find an authoritative source on the limit and have not worked out the math myself yet.) See below - Theoretical foundations

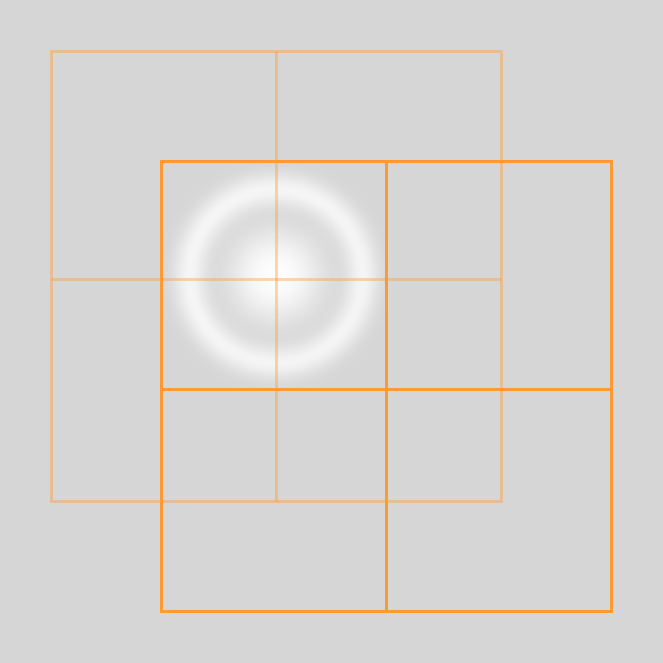
However consider now that we massively increase our sensor resolution (or we can pixel shift to that effect), then we sample the Airy disk so much that we can perfectly reconstruct its true shape. We cannot know however what the true shape of the object is that creates the Airy disk - it might be as small as a single nanometer-sized photon source, but its image is never smaller than this blob of a few microns in diameter.

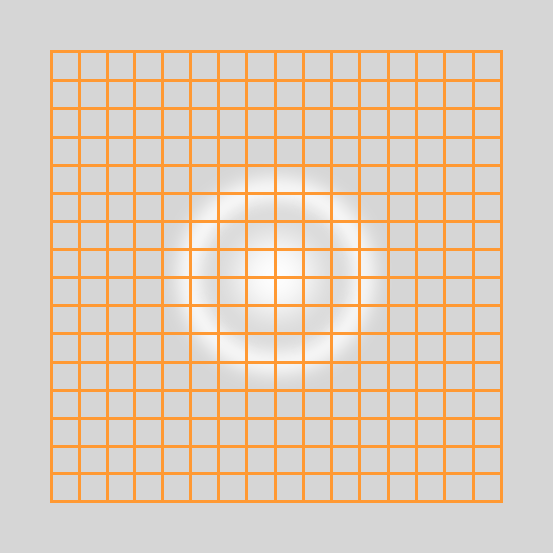
As far as I know for color images, the only possible improvement to the size of the Airy disk for a given lens aperture is in the special situation of transparencies - the size of the airy disk can be decreased by a factor of 2 with a special lighting setup and a bit further using oil immersion - these techniques alter the numerical aperture of the whole optical system, instead of just the lenses'.

See also this question for more insights on the diffraction limit.

Theoretical foundations

(Note: This section still needs work in terms of readability and clarity, but it also has become mostly redundant after finding the wikipedia article on optical resolution)

The topic can be approached from a spectral perspective. Let's initially consider the 1D case.

Every point in the photographed scene can be understood as a single dirac pulse - from a spectral view, a dirac pulse contains all frequencies equally (|F(f)| =const.).

Passing this through an ideal but finite-sized aperture leads to a low pass filtering - the single point gets projected onto a less sharp image we call the Airy disk - whose frequency spectrum follows the square of the sinc function (|F(f)| ∝ sinc^2(f)). So this attenuation of higher frequencies is the fundamental limit in resolution for a given optical setup.

Now add the sensor to the equation. The sensor consists of many rectangular photosites and for modern image sensors let's assume pixel pitch equals pixel size (e. g. 100% fill factor). Each of these squares has a frequency spectrum of $$|F(f)| = \frac{1}{X_{pixel}}sinc(f \frac{1}{X_{pixel}})|$$. However, the spatial frequencies that lie beyond half the pixel pitch will become visible as aliasing in the image - to avoid this, we need to shrink the distance of the pixels down to the point that sufficiently much of the information in the airy spectrum remains in the pass-band of the sensor. Practically however we cannot create pixel pitches smaller than the pixels themselves. They cannot overlap, right?

Enter pixel-shift. With pixel shift, even though the individual pixels stay the same and each individual pixels spectral transform remains the same, taking multiple shots of the same subject with sub-pixel shifts allows us to decrease the pixel pitch beyond the physical distances. If we halve the pixel pitch, we double the aliasing frequency.

If this thought process is correct, we can infer:

• The size of the airy disk limits is a fundamental limit to the resolution.
  The smaller the airy disk, the more frequencies remain.
• The size of the photosites limits the amount of information the sensor can capture.
  The smaller the photosites, the higher the frequencies that each of these can resolve.
• The pixel pitch determines the frequency at which aliasing artifacts occur and therefore how much the aliased content degrades our image.
  The smaller the pixel pitch, the higher the aliasing/Nyquist frequency. The pixel pitch can be decreased by pixel shifting.

So in essence, the frequencies present behind the lens are the result of the interplay between the airy disk given as a function of f-number, the photosite size and the pixel pitch $$ sinc^2(\mathrm{aperture}), sinc(X_{\mathrm{pixel}}), DiracComb(X_{\mathrm{pitch}})$$.

Considering the 2D case, we also have to look at the shape of the aperture and the pixels - to say it in the words of Sepp Herberger: "The round must go in the square", where the round is the image of the aperture - the airy disk and the square is the pixels. But for the moment, this will be left as an excercise for the reader ;)

Answer 3

All camera optics are plagued by twin demons of interference and diffraction. These yield stray light rays that comingle with the image forming rays. Diffraction is caused when light rays from the vista being imaged, just brush by the edge of the aperture stop. Some close passes are shadowed but not completely blocked. The ricochets comingle and degrade the image. Interference is due to the wave nature of light crossing paths and adding and canceling each other.

Well studied by Lord John Rayleigh, Astronomer Royal British 1842 ~ 1919 Nobel Prize physics 1904.

His calculations, remain valid. We are talking about the resolving power of a lens system. Following is a table for 589, about the center of our color spectrum.

Called the Rayleigh Criterion (to my knowledge, never exceeded)

f/1 1392 lines per mm

f/2 696 lines per mm

f/2.8 487 lines per mm

f/4 320 lines per mm

f/5.6 249 lines per mm

f/8 184 lines per mm

f/11 127 line per mm

f/16 87 lines per mm

f/22 63 lines per mm

f/32 44 lines per mm

Answer 4

Yes but you are trading one type of information for another - it doesn't break the laws of physics or information theory. You have to assume the object is stationary and you are trading signal to noise for resolution.

There are many possible approaches. One is simply blocking out the centre of your optical system and only using the edges. The central peak of the transfer function of this is narrower than for a circular aperture so your resolution is increased but you both have less signal received and have wider wings in the transfer function, both reducing signal to noise.

Answer 5

Several post-processing techniques that increase the resolution limits of a camera/lens system can be used to ameliorate the effects of diffraction. Stacking multiple images taken from slightly different positions as you suggest is one way. A tool such as Canon's Digital Lens Optimizer that uses very detailed lens profiles is another.

Diffraction is a lot like the edges of depth of field. The more we magnify an image the easier it is to see. Diffraction starts at apertures where only very high magnification will reveal any effects at all. As the aperture is closed down further the effects begin to be perceptible at lower and lower magnifications.

What we often refer to as the Diffraction Limited Aperture (DLA) for a specific digital sensor is the aperture at which the effects of diffraction are noticeable when the resulting image file is viewed at a magnification that yields 1-pixel in the image file equals 1-pixel on the monitor and those individual pixels are right at the limits of the viewer's perception to differentiate them. The DLA is the point at which the effects of diffraction are barely perceptible at such a magnification. This begins to occur when the size of the blur caused by diffraction becomes larger than the size of a digital camera's sensor pixels.

What we refer to as the Diffraction Cutoff Frequency requires a much narrower aperture setting than the DLA for a specific sensor (or film - the size of the grains in various films affects the DLA with film!).

For more on how the DLA is affected by the resolution limits of the recording medium, please see: Does sensor size impact the diffraction limit of a lens?