How much zoom would make a camera suitable for imaging railcar seals from 100 feet away?

Question

I'm an engineer, and we have a need to take pictures of railcar seals (steel ties as pictured) from on top of the building. What I need is a camera with sufficient zoom so we can clearly see whether the cap is correct and the tie is intact (if the writing could be legible that would be a bonus, but I'm not counting on it). The longest distance I expect to snap the shot from would be 96 feet (normally more like 30 feet but that's a worst-case scenario). The tags are about 1 1/16" long. The engraved tags will probably only be legible from up close I would think. If the tags themselves are not possible at these distances, it might be enough just to confirm that the cable is intact.

Would a so-called superzoom camera be sufficient? If so is 24x too small or do we need to pick up something closer to 60X? Photos would be taken during the day facing east (polarizing filter to reduce glare?). Our rail manager will be taking the photos and is an amateur, so point and shoot capability would be helpful.

Bad image taken from side of car

We haven't decided on a budget yet, but less than 700 would be nice.

Answer 1

As the other answers do a good job of giving you the fish, seeing as you're an engineer, this answer will teach you to fish.

The angle of view of a lens & camera combination is simply determined by the isosceles triangle whose base is the diagonal of the camera's sensor size, and whose height is the lens's actual focal length. The vertex angle of the isosceles is the angle of view.

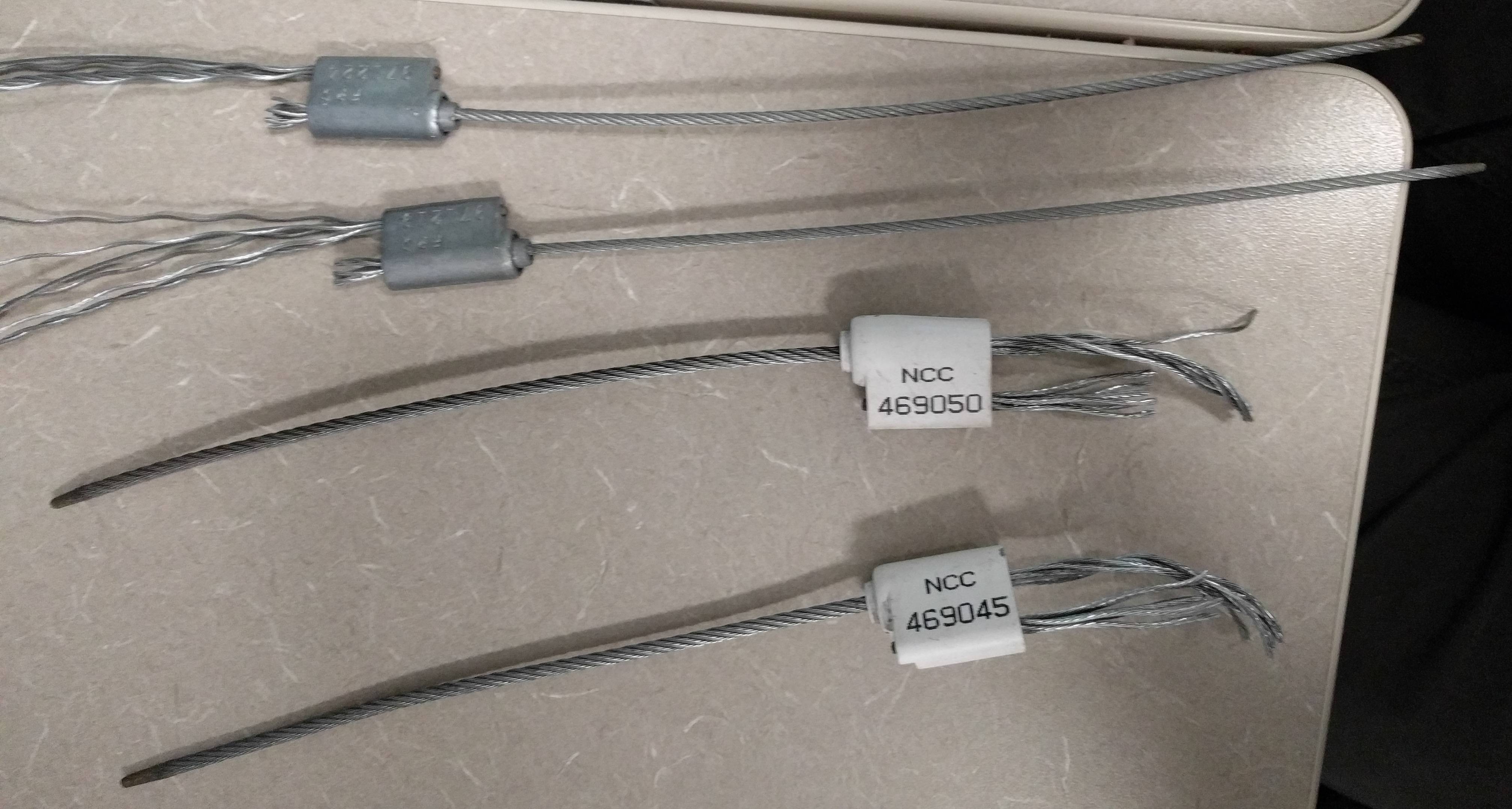
We could actually calculate the desired angle of view using trigonometry, but let's just do some calculation based on similar triangles. Assuming the image you included is roughly the size you need to be able to read the tags, I measure the tag at about 1/12 the width of the image. So the image is 12 3/4" wide. Let's truncate to 12", or 1 ft. Then your subject distance:width ratio (D/W) is 96:1 (in your stated worst case). Using the distance as the height of a isosceles triangle, and the subject width as the base of the triangle, we have put bounds on the angle of view.

In the image below, the gray box represents the camera. D is the distance to the subject (i.e., 96 ft), and W is the width of area to cover (12 inches). Because the triangles are similar, ƒ/d = D/W.

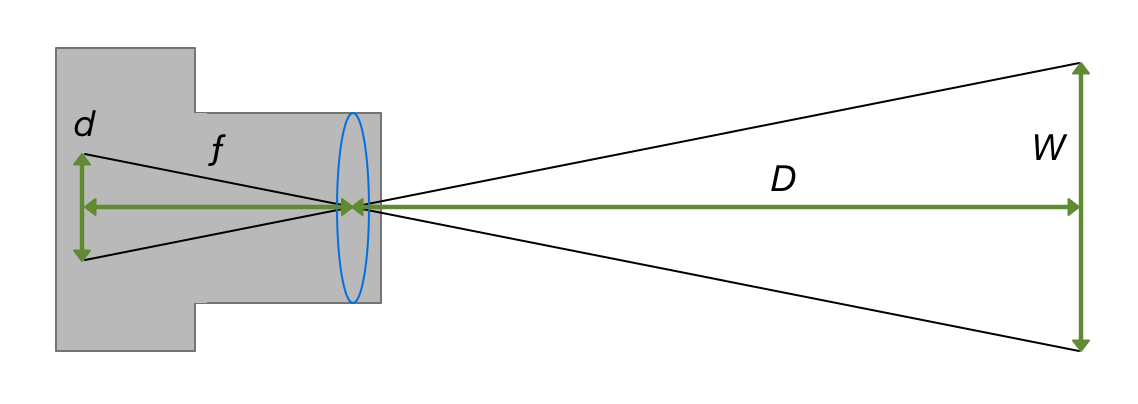
Therefore, by similar triangles, your camera's focal length–to–sensor diagonal ratio ƒ/d should meet or exceed 96:1.

AJ Henderson's answer suggests a Nikon P900 superzoom, which has a "35mm-equivalent" focal length of 2000mm. The sensor size of a 35mm format sensor is 24mm × 36mm, so the diagonal is ~43mm. This gives a ƒ/d ratio of about 46.5:1, or around half of what you would need to produce a similarly size image as you provided, at 96 ft.

Note: the P900 doesn't have a 35mm-format sensor (it's actually 6.2mm × 4.6mm (7.7 mm diagonal)), but since the focal length / angle of view is given relative to a 35mm sensor size in this discussion, we use a 35mm sensor as the calculation baseline. The actual physical max focal length is 357mm (which is 2000/357 = 5.6 times smaller than its "35mm equivalent" focal length). But since the camera's sensor is 5.6 times smaller than a 35mm-format sensor, the scale factors cancel.

---

So, let's step back. The best focal-length–to–sensor-diagonal ratio you can expect with the P900 is 46.5:1. Is that enough for you? That translates to an image that covers about twice the width of the image you provided, at 96 ft. Put another way, from 96 ft away, a P900 at full zoom will produce an image where the seal tags will be about half as wide as the ones in your example image. Is that adequate? Make sure you consider AJ Henderson's caution about motion blur: you will have to make sure the camera is steady in order to not have blurred details at that level of zoom.

I suggest to rent a P900 and test it, before you commit to buying. For around $60, you can rent one from places such as LensRentals.com or BorrowLenses.com for a week.

Answer 2

Make some tests using your existing camera. This is providing you can glean the focal length setting used for the exposure. Take a series of pictures at measured distances. From this test, decide the maximum distance and focal length used that delivered a satisfactory image.

Suppose the image taken from 12 feet with a 300mm lens focal length is satisfactory. Now you can calculate that a subject distance of 24 feet taken with a 600mm will yield a satisfactory image. Continuing – 48 feet with a 1200mm ; 96 feet with a 2,400mm; etc.

Note: 24X or 60X can be deceiving. These are zoom ranges. You need to look at the actual focal length the zoom lens delivers. After running the above test, you can determine for yourself the needed focal length. Additionally, all lenses are not created equal. You get what you pay for.

Answer 3

The Xtimes zoom designation is useless here. It means that the ratio between viewfields / focal lengths of full zoom-in and full zoom-out is X. You don't know whether it can shoot fish-eye images covering 120° angle when zoom-out or it can see windows on a plane 4000 ft above when zoom-in.

The key factors for your consideration are: resolution, sensor size and focal length. Resolution is self-explanatory, focal length determines how big the projection of the tags will be on the sensor and the sensor dimensions rule how many pixels will cover the tag.

In short: The bigger resolution, the better. The longer focal length, the bigger the tag. The larger sensor, the smaller the tag.

Say we want to have 10"-16" viewfield. Using this online calculator and this table for crop factors we can get focal lengths you need to cover:

• For APS-C sensor used in entry level DSLRs and mirrorless cameras you would need to cover 800 - 2500 mm.
• For 1" sensor used in Sony RX series, for example, you will need to cover 400 - 1200 mm.
• For 1/1.7" sensor used in high-end compacts you will need to cover 250 - 900 mm.
• For 1/2.3" or 1/2.5" sensor used in superzooms you will need to cover 200 - 600 mm.
• For APS sensors used in full-frame professional DSLR/mirrorless you would need to cover 1200 - 3600 mm.
• For medium frame used in ultimate art studio cameras you would need to cover 1500 - 5000 mm or 2000 - 6000 mm.

Superzooms are probably out because they provide fixed range of focal lengths possible and the range is decided by the manufacturer to cover what Average Joe would need.

Full frame and larger are out because of high prices. They are pro- and ultimate- kits; I suppose you need to evaluate the seal condition and read the tag.

I would recommend to look for entry-level DSLR or mirrorless body equipped with teleconverter ring and long lens. If you get 2x teleconverter you will be OK with 400 - 1200mm lens, with 4x teleconverter you will be OK with 200 - 700mm lens.

If you can sacrifice the details, you can go for shorter lengths, since the seal would cover smaller part of the image.

---

Regarding the comments: Your Nexus 5X is equipped with 1/2.3" sensor. Supposing the 26mm value is shortest focal length of the lens. In that case you need 23x zoom (600 / 26 is roughly 23).

Answer 4

After giving this some more thought and research, I believe what you are trying to do may be possible, but it may be difficult and will require zooming in on the image to be able to check.

My initial answer was ignoring the fact that at modern camera resolutions, the seal can be relatively small in the frame and still be able to be recognized as being intact.

I do expect that camera shake will be a significant challenge at the levels of zoom you are talking about, but a camera like a Nikon P900 has a 35mm equivalent focal length of 2000mm with a digital zoom option to go to 4000mm which might be enough to give you what you need even without cropping.

It is still probably a good idea to get some type of a tripod with that could be used for steadying the camera when taking shots from 96 feet, but the P900 does have fairly good image stabilization built in that will help.

I wouldn't plan on being able to read the tags, but there's a decent chance the seal itself will be visibly intact.

Answer 5

I have the Nikon P900, and its effective 2000mm focal length is what you want. At 30 meters with a 357.5 mm focal length the area you take a picture of is 386 by 520 mm or about 15 by 20 inches. This is a little larger than you want, but close. At maximum zoom it is hard to get it pointed where you want, but there is a button on the lens that zooms out to show the general area with a box showing what will be in the frame when you release the button and it zooms back in. With practice you can hand hold it and get sharp pictures, but a tripod makes that much easier. It has a birdwatching mode that tries to keep the shutter fast by raising the ISO value. That makes a bit more noise but fights blur from camera motion if you are hand holding. If you get good focus I would expect to be able to read the tags.