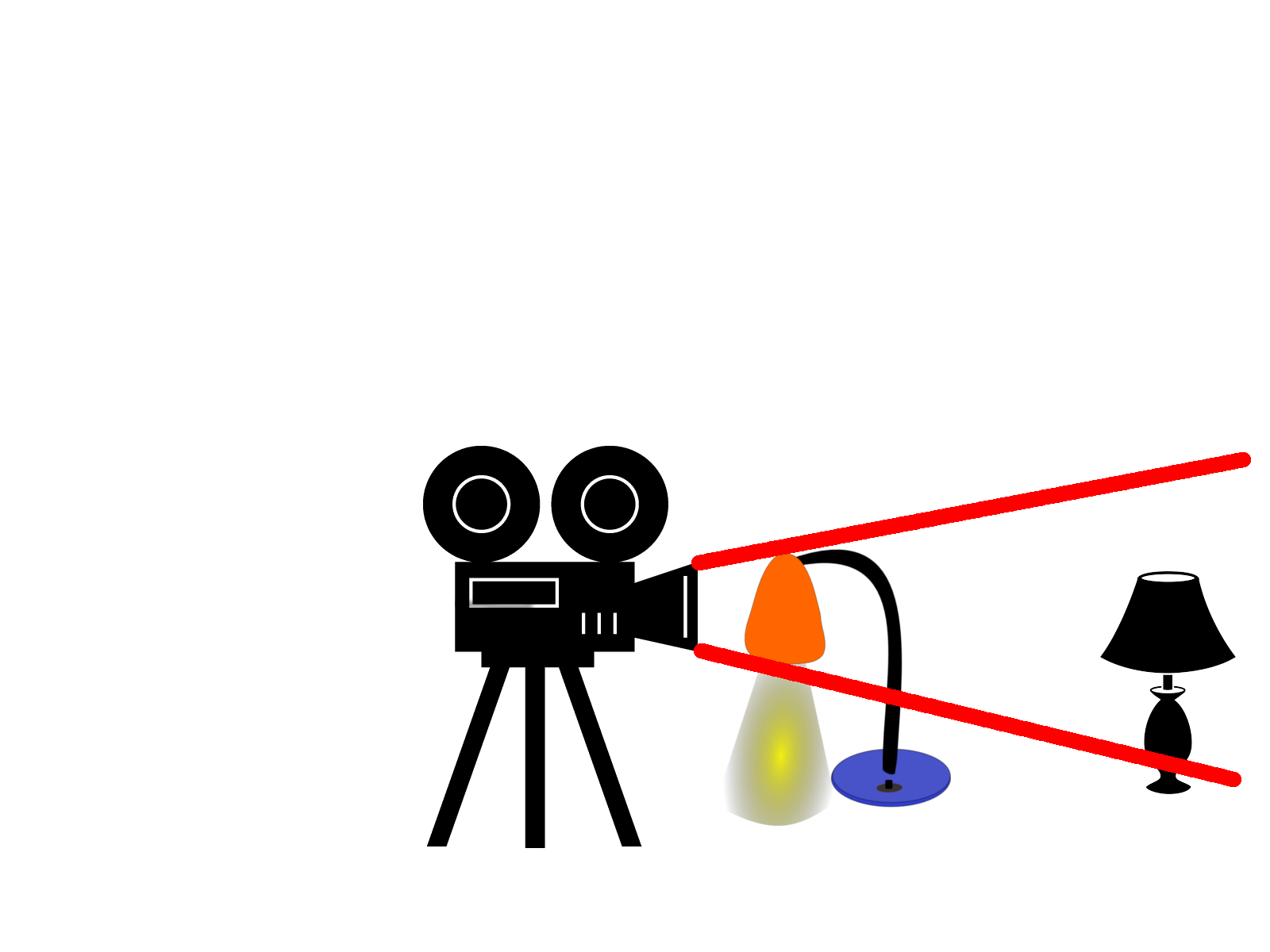
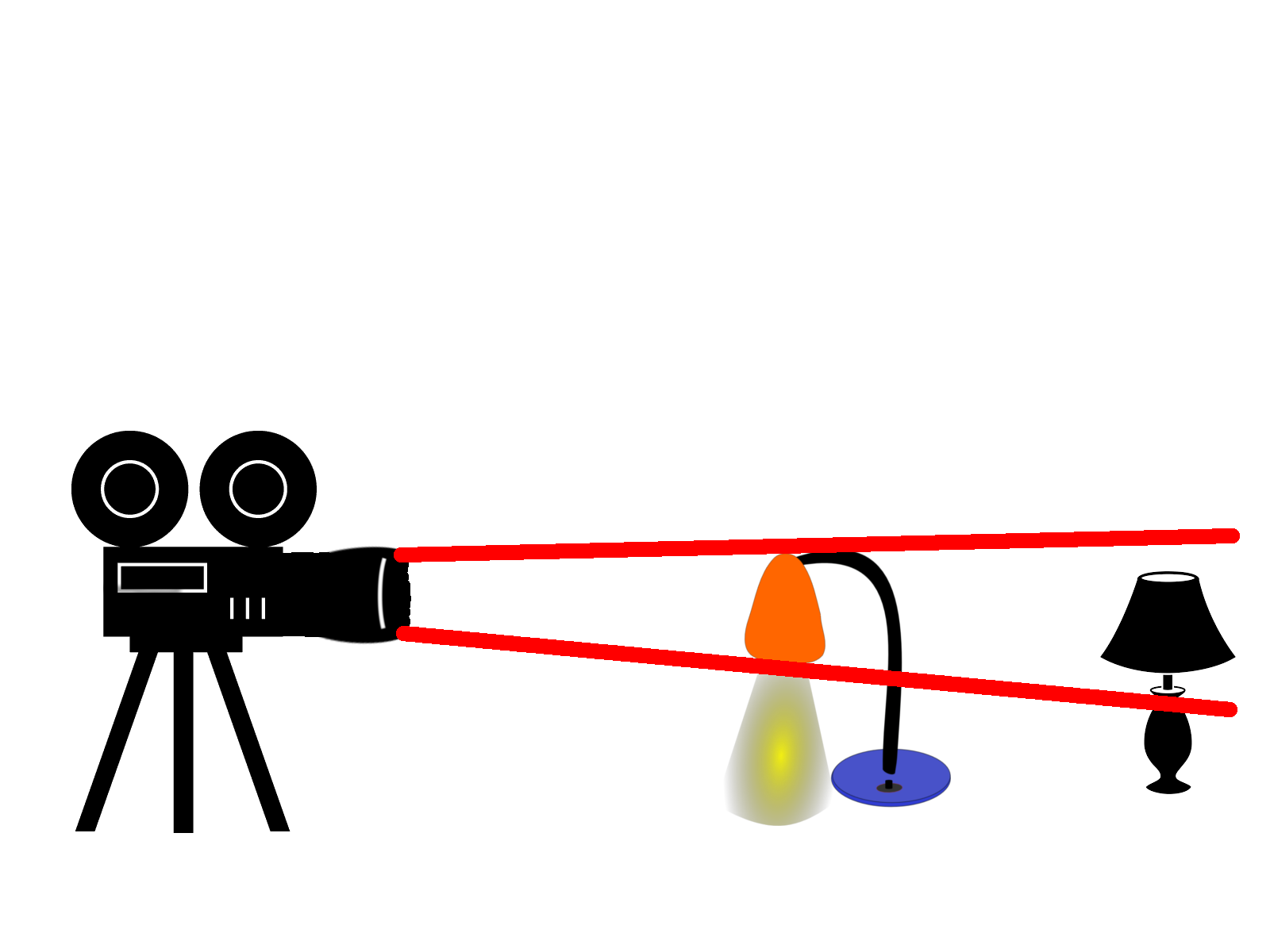
The differences between the two images are caused by what is known as perspective. The basic idea about perspective is that as the camera position is changed the angles and relative distances between various objects in the scene will change as well. Focal length only comes into play because we use a different focal length to make a subject the same size in the photo when we have changed the subject distance or conversely we change the subject distance to accommodate the difference in focal length. If you use a wider lens and just crop the result it has the same effect as using a longer focal length when both lenses are used from the same position.
If you have to move further back with the 25mm lens to make the lamp the same size in the frame as it was with the 20mm lens then you've changed the distance to the subject by increasing it 25%. But you haven't increased the distance to the background by 25%, because it was further away than the lamp to begin with. So the same increase in feet is a lower percentage of the distance to the wall in the background than it was as a percentage of the distance to the lamp.
Let's say for simplicity's sake the lamp was four feet from the camera and the wall was 16 feet behind the lamp and thus 20 feet from the camera when the shot was taken with the 20mm lens. Now with the 25mm lens the distance to the lamp is five feet, an increase of 25% which perfectly offsets the 25% increase in magnification of the 25mm versus the 20mm lens. But the distance from the lamp to the wall has not changed, it remains 16 foot. This means the distance to the wall from the camera has only increased from 20 to 21 feet, an increase of only 5%. Because we used a lens that increased magnification by 25% and only increased the distance to the wall by 5%, the wall will be magnified more in the second image and will thus appear larger. To make the wall the same size in the image using the 25mm lens as in the image shot with the 20mm lens the distance between the lamp and wall would need to be increased from 16 to 20 feet which would thus increase the distance from the camera to the wall from 20 (4+16) to 25 (5+20) feet or 25%.