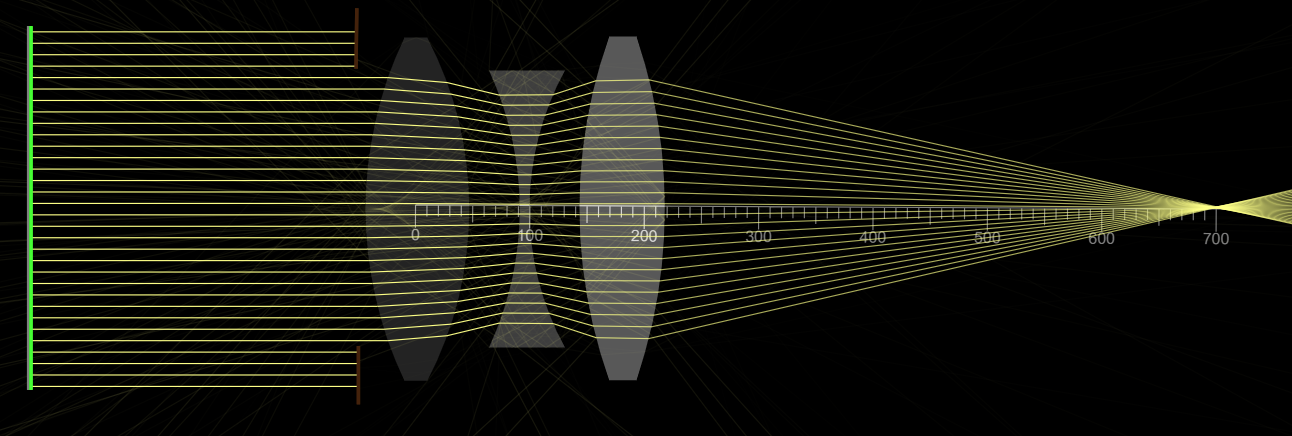
I've heard of three common ways to compensate for spherical aberration:
- Small aperture (fewer non-paraxial rays).
- Aspheric lens (compensate with lens shape/material).
- Corrective spherical lens elements (?).
I have a rough understanding of the first two, but I don't understand the third. How does using more spherical elements correct spherical aberration?
Also, do these extra spherical elements just reduce the circle of least confusion or do they fully correct the aberration? It doesn't seem possible to me that you could fully correct spherical aberration by only using additional spherical lenses.