Can an RGB channel be shifted to increase color gamut into the violet range?

Question

TL;DR: Can additive RGB color gamuts produce the perception of light that has a shorter wavelength than the Blue component? If not, does shortening the wavelength of the shortest component of a 3-channel-color system (e.g., "Blue") increase the range of human-perceptible colors producible by the gamut? Is that not done due to technical obstacles?

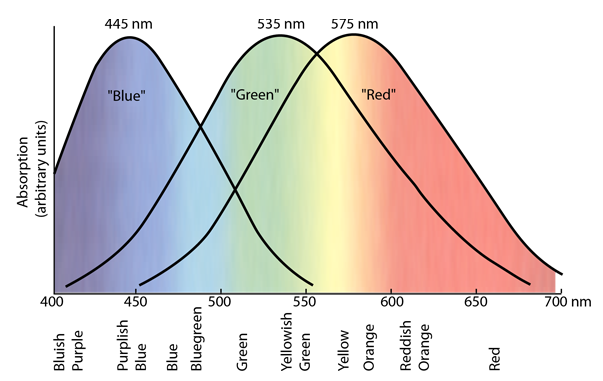
Digital photography and light projectors typically use an RGB (Red + Green + Blue) color model, which at least superficially makes sense because it approximately corresponds to the peak responses of the three human color receptors:

But humans can perceive light with higher frequency than "blue." Spectrally we refer to that higher-frequency color region as "violet" or purple:

It is not physically possible to produce light with a frequency higher than the Blue component through any combination of RGB color projectors.

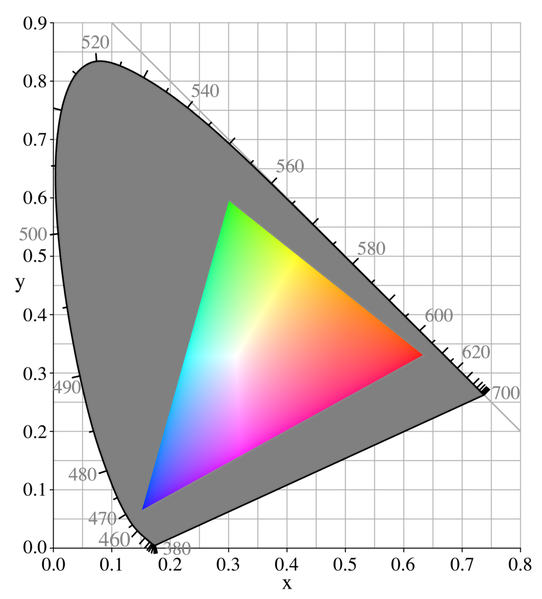
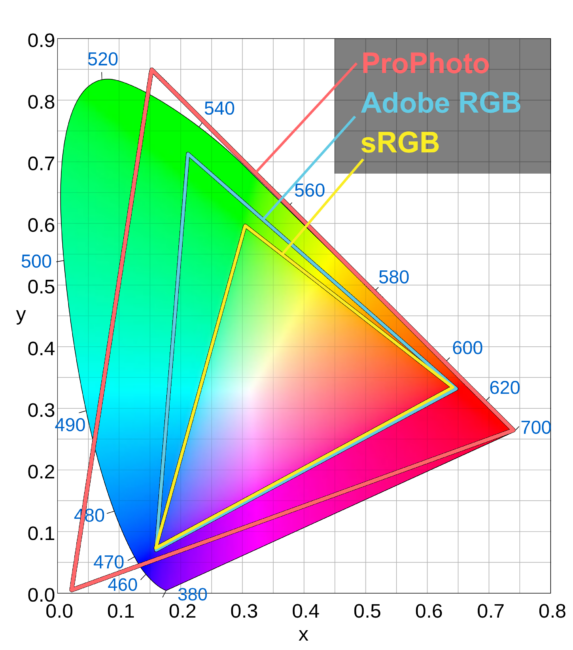
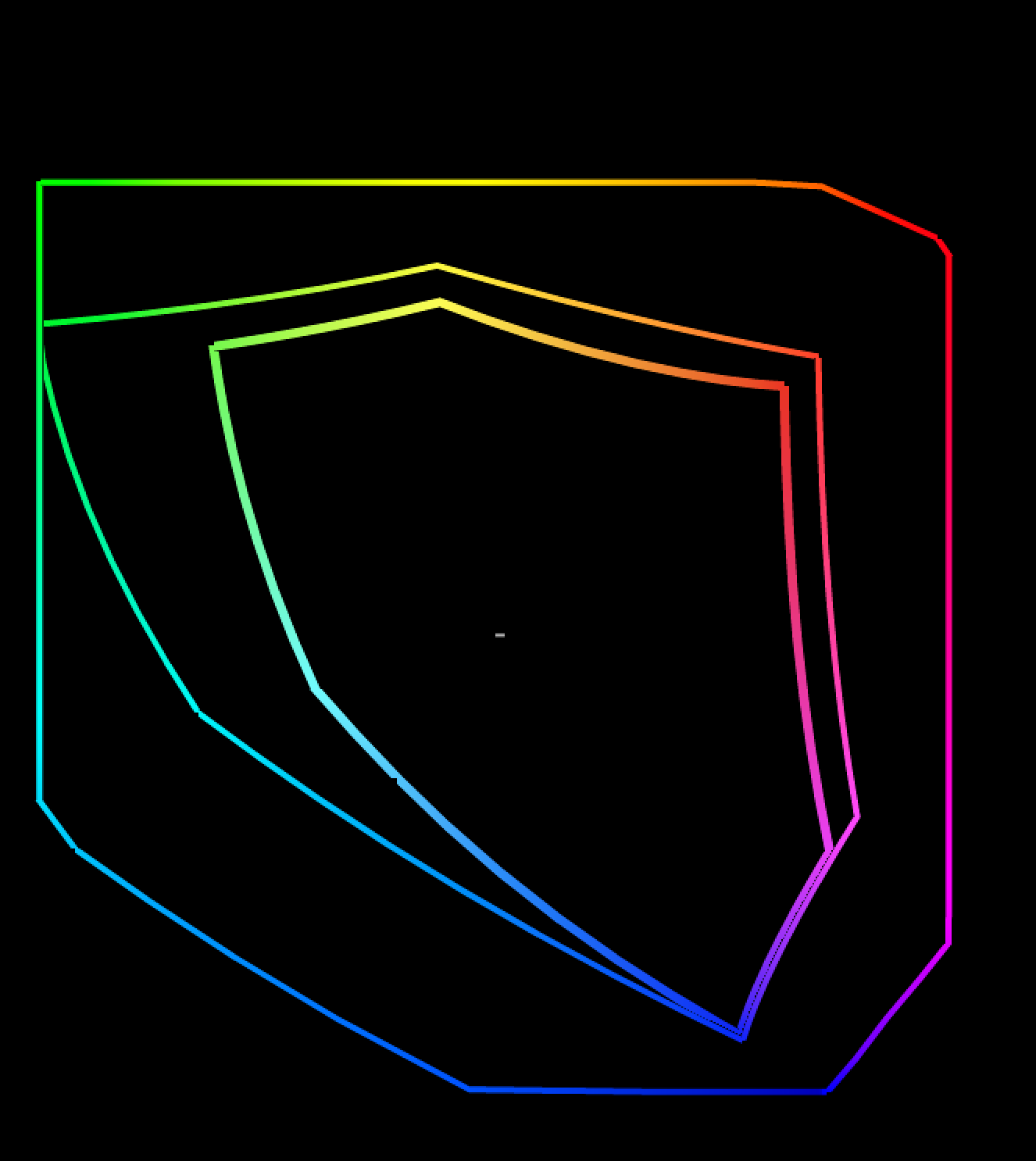
We know that a standard RGB color gamut does not cover the range of perceptible frequencies. Here is a standard gamut coverage chart, with the gray area indicating "colors" that cannot be produced by any combination of the RGB source:

Considering the violet region: There is a region in the RGB gamut we refer to as "violet," which is a combination of red and blue. But referring back to the photoreceptor response curves it is distinguishable from true violet because true violet barely stimulates the red photoreceptors.

If we increase the frequency of the highest-frequency component of a 3-color projector – i.e., we increase the frequency of "blue," pushing it towards the "violet" limit of human color perception – do we not increase the coverage of the projector gamut?

I suspect the answer is "Yes. But: the blue photoreceptors are not excited as strongly by the violet frequency. The RGB frequencies were picked to correspond with the peaks response frequency of each photoreceptor. If you shifted the blue frequency towards violet (call this an RGV gamut) then your projector would have to be able to output more violet light than the red or green channels in order to cover the rest of the unshifted gamut." If that's correct then this is a technical issue, and not one that seems particularly challenging. For back-lit projectors, which produce color by filtering a white source, the red and green filters would have to be recalibrated to cut more light than the violet filter.

However the answer might be, "No: See that region between blue and green? No matter how you boost your boosted violet channel it just can't reach into it as far as a centered blue channel." (I think the only way to reach this answer would be to have complete parameters of the photoreceptor response curves to spend some time linear programming.)

Answer 1

Yes but you do not even need to.

The color gamut indicated by the triangle is the coverage of linear combinations of the three RGB primaries. By moving the primaries you can expand or contract the color gamut. This can be seen if you compare the sRGB and Adobe RGB color spaces, both are RGB with slightly different primaries.

Theoretically, you can move the primaries anywhere. The reason though they are not simply moved really wide apart is to avoid banding due to quantization. While mathematically any color within the color-space is part of it, when using fixed color-depth, not all exact colors are representable. So a wider color space has larger steps in between colors and therefore is more likely to show banding and other color artifacts.

Given modern advances, one can use deeper pixels and even floating point respresentation which allows for a wider color-space while minimizing quantization errors. Take a look at the difference with ProPhoto RGB that does something similar to what you suggest:

Source Photography Life

Once colors can be represented by non-integers, the next step is to allow negative values. This is easy to store in floating point numbers but is also used with fixed-point numbers. The sRGB64 color-space, now renamed scRGB, does exactly this and achieves an extremely wide color-gamut that covers nearly the entire visible light spectrum because each component can from around -0.5 to 7.5 as a factor multiplying a primary. See the diagram in the Wikipedia articled.

Answer 2

Gamut is much different than sensor spectral sensitivity. The title of your question infers gamut so to start there, digital cameras do not have gamuts.

Color gamuts are applied to sensor data when pixels are made and a container color space is chosen. So the gamuts shown are limited by the RGB color space not the camera's sensor.

So to achieve the largest gamut from any sensor data I would recommend using ProPhotoRGB as the destination color space in your raw file conversion process. Change the destination color space to ProPhotoRGB a significantly larger gamut than what is in your graphs.

How does this happen? Well The ICC profile is a container space defined in XYZ and converted to CIELab. RGB is static and all RGB color values are limited to 0-255 for each channel. The code values though are determined by the limits of the container space in CIELab.

If the first graph is an indication of direction towards spectral sensitivities, then those are determined at the time of sensor creation. The RGB filters used over the sensor attempt to get as close to human cone responses as possible in the world of filter technology.

So if you are attempting to maximize something different than gamut, I would have to ask for what purpose? This is an important question because there are many solutions beyond the standard sensor technology and capture systems, but they are all solution specific.

Photography is art and is sold as art. So much of what we can do technically can either enhance that art, detract from it, or make it cost-prohibitive.

Multispectral Prism cameras are still part of the latter group.

Answer 3

Apparently our color perception is willing to be tricked into perceiving "violet" using only lower-frequency wavelengths. I finally found the following explanation of the phenomenon (reproduced at length here because Quora does not preserve content):

Monitors don’t deliberately output light in the violet frequency range. Even so, they can produce what we see as violet light.

The reason for that is that the frequency of light that we call “blue” actually stimulates “blue” photoreceptor cone cells in our eyes that, when stimulated by themselves, alone, give us the sensation of seeing violet — not blue.

We see blue because blue light also triggers green cones, and the combination of these two types of cone cell firing in the associated particular ratio gives us the sensation of seeing blue.

There are two separate ways to get the “blue” cones to effectively fire by themselves, producing the sensation of seeing violet.

One way is to use violet light. This stimulates the “blue” cones without stimulating the red or green cones very much. Monitors can’t normally do this, because they are built with red, green and blue pixels.

Another way is to use blue light, but add in some red light.

Take the combination:

(some red light) + (much blue light)

The red light stimulates mainly red cones, while the blue light stimulates mainly green and blue cone cells.

As far as our eyes are concerned, we then have all three types of colour receptor firing, plus the blue cones are firing even more than the others. The combination of all three is perceived as white and can be simply filtered out by our brains. What’s left over is as if only the blue cones were firing, and that gives us the sensation of seeing purple.

This is also why, even though visible light lies on a spectrum from red through to violet, it appears to us as more like a circle, with violet fading back into red. The addition of increasing quantities of red light to blue light produces first the sensation of seeing violet, then later a more reddish hue as we continue to add red, until finally we are completely back to red.