OK, trying not to sound like I'm promoting a product here, but yesterday I discovered a free online tool that visually answers my question in the manner that I was thinking about when I first asked it. I am a paying customer of another tool by the same author, but this answer is not an endorsement of that paid product. I'm also keeping Osullic's answer as the selected answer, as that answer does numerically explain what I am asking, and this answer simply confirms it via a visual method.
The tool I discovered yesterday was a visual Field of View calculator produced by Crookneck Consulting (whose main product is the Photographers Ephemeris). This tool allows the user to visualize the frustum of different camera sensor formats and lens focal lengths when viewing a simple 3D model of a tree (24m high) that is a fixed distance (100m) from the camera (that is 1.6m above the ground). In this way you can visually see how the scene will fill the sensor.
Note that the numbers produced by this tool are slightly different to FoV calculator that Osullic used. I don't know why that is, but I am assuming that configuration changes within the same tool produce numbers that are representational to the changes themselves. So I am not so worried about exact numbers as I am to the relationship between the numbers.
As an example, here are some screen shots of different camera formats and lenses.
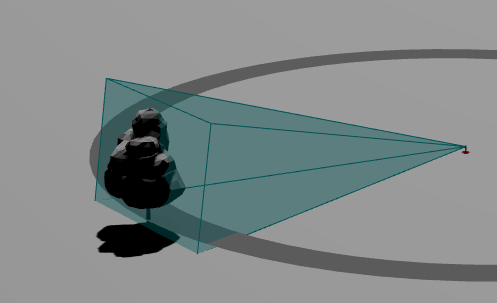
The first is for my Nikon D7500 (DX sensor) with a 50mm lens and is pitched up 8 degrees so that the lower part of the frustum starts at the base of the tree. The tool calculates a horizontal FoV of 26.7 degrees and a vertical of 18.1 degrees.
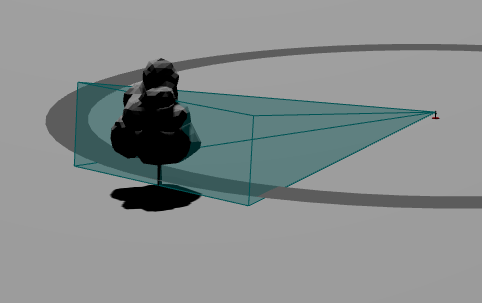
The second format is a 6x17 sensor with a 105mm lens pitched up 15 degrees. This is the configuration of the Fuji GX617 that I am considering. The horizontal FoV is 78.0 degrees and a vertical is 31.9 degrees. This has a much larger FoV than the previous 50mm lens on my Nikon. For the same vertical FoV on my D7500, the equivalent lens would be 28mm.
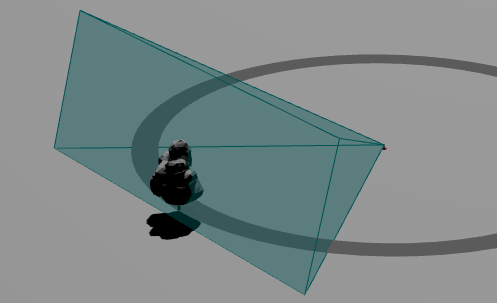
The third one is the same GX617 format, but with the 180mm lens, pitched up 9 degrees. The horizontal FoV is 50.6 degrees and the vertical 18.9 degrees.
This image shows that the tree would vertically fill the GX617 negative by roughly the same proportion as my current D7500 and matching 50mm lens which has a vertical FoV of 18.1 degrees.
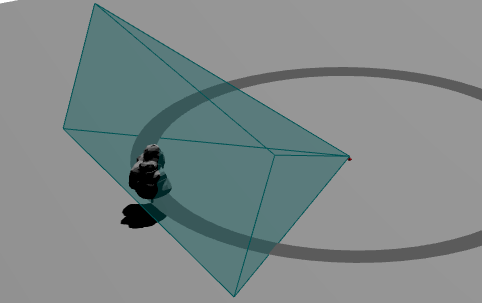
The is the GX617 300mm lens pitched up 5 degrees. The horizontal FoV is 31.5 degrees and the vertical is 11.4 degrees. For the same vertical FoV on my D7500, the equivalent lens would be 80mm.
Finally for completeness, the GX617 95mm lens, pitched up 17 degrees. The horizontal FoV is 83.6 degrees and the vertical is 35.1 degrees. For the same vertical FoV on my D7500, the equivalent lens would be 25mm.