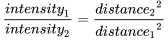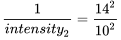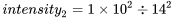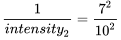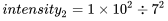@BobT made a good point about the effect of shutter speed (or exposure duration).

Light fall-off and the Inverse-Square Law

There is another aspect that hasn't been mentioned in the responses ... and that's the topic of light "fall-off".

The amount of light that lands on a subject change based on the distance between the light source and the subject. The change is based on the inverse square law.

Camera lighting is usually not the only source of light for a scene. There usually are some sources of ambient light. You can think of the ambient light as continuous lights. The longer the shutter remains open, the more continuous light will build up on your subject. Photo strobes, on the other hand, are not continuous lights. Your camera shutter opens, the strobe fires, and regardless of how much longer the shutter remains open it will not receive any additional light from the strobe.

Suppose you get an acceptable exposure without flash as long as the shutter is open for a long enough period. You decrease the duration of the exposure (which results in less ambient light) but you supplement with a photo strobe. If the power on the photo strobe was set to allow for a correct exposure of the intended subject, you may notice that anything in the scene nearer to the camera is especially bright and possibly over-exposed. Meanwhile, things in the scene located farther from the subject will appear much darker and possibly black.

These two photo examples (the "with" and "without" flash) wont actually resemble each other at all. The supplemental light changes the ratio of light from ambient (continuous light) sources vs. strobes (momentary sources).

In general, if you place a light source and set its intensity to correctly illuminate your subject at some distance, then you can calculate the amount of light that will illuminate other elements of your scene based on their distances from the same light source using the relationship described in the formula below.

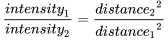
For example, suppose you have a photo of a person who is located 10 feet away from the light source. There is a person a bit nearer to the camera and they are located 7 feet away... and another person located 14 feet away. Here is what would happen:

In the first instance where a 2nd subject is located 4 feet farther away (14 feet from the light) the equation looks like this (I'll use the value of 1 for the correct amount of light intensity because it makes it easier to see what the intensity is of the other subjects):

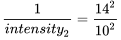
This is solved as 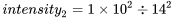which works out to .51 ... so the person located 14 feet away would get only about half as much light as your main subject.

Meanwhile another person located 3 feet nearer than your intended subject looks like this:

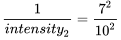
This is solved as 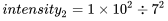which works out to 2.04 ... so the person located 7 feet away would get twice as much light as your intended subject. Also note that means that the person 14 feet away is only 1/4 as bright.

This is what is meant by the light fall-off problem.

You can decrease the severity by moving the flash farther away. So imagine the subjects are all still 7, 10, and 14 feet away from the camera location ... but the strobe is located 20' away from the main subject. So the distance from the light source to your subjects is now 17, 20, and 24' away.

If we assume we have the light adjusted to properly illuminate the subject at the 20' distance then

... the person at the 17' distance receives 1.38 times more light (a little more than a third brighter)

... the person at the 24' distance receives .69 times as much light (about a third dimmer)

And those differences aren't too bad. This assumes the light source and any modifiers (reflectors, soft-boxes, etc.) can adequately light the subject(s) at those distances.

(On a side-note, Photography StackExchange doesn't support LaTeX (some other StackExchange communities do) ... so the equations had to be generated as .png files and imported as images.)

Noise Differences

There are many kinds of noise. But generally the type that dominates most images tends to be read noise. The amount of "read" noise in an image is fixed. Extremely long exposures can result in a build-up of heat and that introduces a new type of noise ... but for purposes of this answer I'll assume we are discussing exposures limited to a few seconds ... rather than several minutes.

Noise becomes noticeable when signal is insignificant (e.g. under-exposure). When the signal is boosted to compensate for under-exposure, the noise is boosted as well. Essentially if the image had a poor "signal to noise ratio" (SNR) then boosting the signal will boost the noise and now the noise is noticeable. If the signal was already sufficient (not an under-exposure) then it doesn't require boosting and this means noise isn't boosted ... so it isn't noticeable (it is there ... but you probably wont see it).

This means discussions of whether or not there are differences in noise are really discussions of whether the two exposures had a different SNR.

If exposure 1 was adequately exposed but did not use flash (or other supplemental lighting ... only already present ambient lights) and if exposure 2 was half as long (not a sufficient exposure based on ambient light ... but supplemental light was added to compensate) then those two photos could have the same SNR ... which means there would not be a different in noise.

Noise is mostly the result of insufficient exposure. Keep in mind that ISO in digital photography is a gain applied to the image but... this gain is not applied under after the shutter closes and the exposure is complete. It is convenient to discuss ISO as if it were part of exposure, but it technically a post-exposure process and not part of true exposure. In other words, boosting ISO to compensate for lack light isn't really an increase in true exposure. When I mention that noise is generally the result of insufficient exposure ... it means I'm not counting the ISO boost as a true part of the exposure (and it also explains why photos taken at high ISO seem to have more noise. They have the same amount of noise ... it's just that the noise was amplified to make it more noticeable.)